By changing variables, integration can be simplified by using the substitutions x=a\sin(\theta), x=a\tan(\theta), or x=a\sec(\theta) Once the substitution is made the function can be simplified using basic trigonometric identitiesF = symsum(f,k) returns the indefinite sum (antidifference) of the series f with respect to the summation index kThe f argument defines the series such that the indefinite sum F satisfies the relation F(k1) F(k) = f(k)If you do not specify k, symsum uses the variable determined by symvar as the summation index If f is a constant, then the default variable is xIf you just want to show it converges, then the partial sums are increasing but the whole series is bounded above by 1 ∫ 1 ∞ 1 x 2 d x = 2 and below by ∫ 1 ∞ 1 x 2 d x = 1, since ∫ k k 1 1 x 2 d x < 1 k 2 < ∫ k − 1 k 1 x 2 d x Share Improve this answer
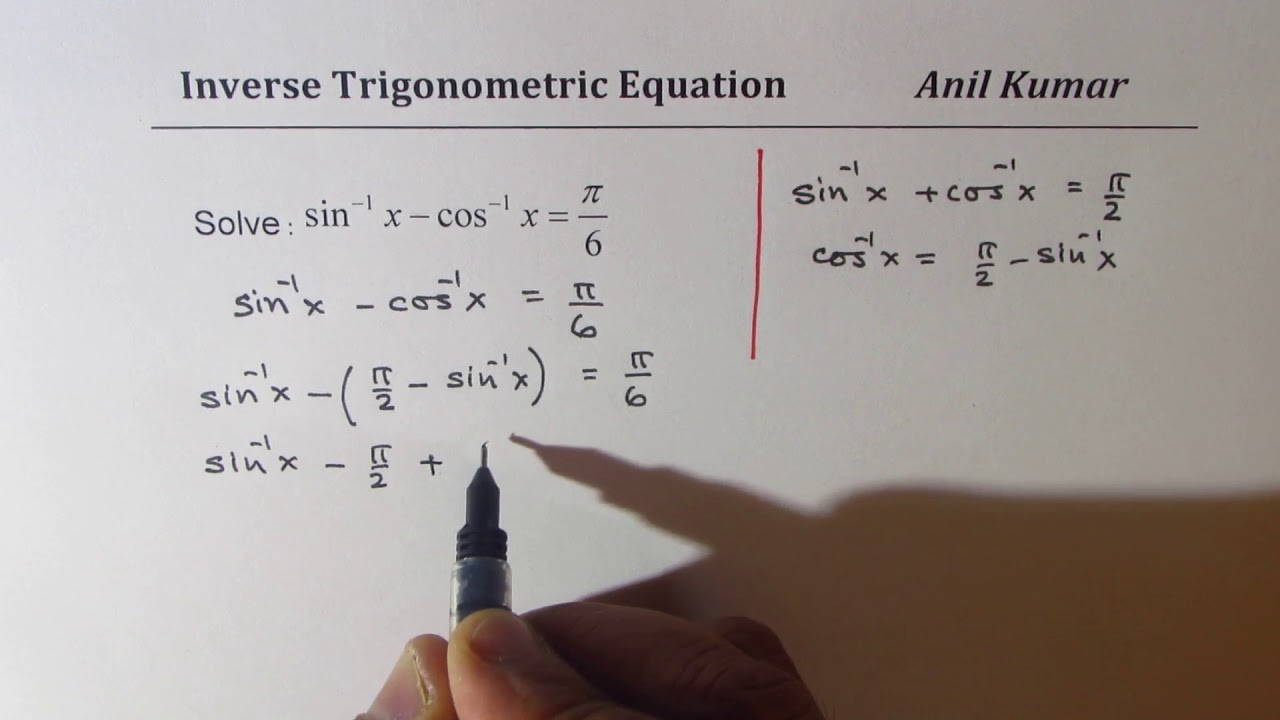
Arcsin X Arccos X Pi 6 Inverse Trigonometric Equation Youtube
Nilai cos 2 π/6
Nilai cos 2 π/6- Title Many proofs that $\sum_{n=1}^{\infty} \frac{1}{n^2} = \frac{π^2}{6}$ can be found in the conformal invariance of planar Brownian motion matr Nome e COGNOME ECONOMIA POLITICA I (San Benedetto del Tronto) Esame del ONLINE Tempo2 ore Esercizirisolvere i seguenti problemi scrivendo le soluzioni esclusivamente all'interno degli appositi spazi




Pdf Another Elementary Proof Of Sum N Ge 1 1 N 2 Pi 2 6 And A Recurrence Formula For Zeta 2k
π2 6 = 1 12 1 22 1 32 1 42 ··respectively †51 Example 37 (Fourier coefficients) Let f be the 2πperiodic extension of the function F −π,π) → R defined by F(t) = ˆ 1 if t ≥ 0, 0 if t < 0 Calculate the Fourier series expansion of f What is its value when t =Oneindige getallen • Topologisch limiet → ∞ • Algebra¨ısch bestaat niet (∞−1=?ofwel ?1=∞) • Maat voor grootte van verzamelingen kardinaalgetallen als ℵ0 • 'Maat' voor ordeningen ordinaalgetallen als ω en 0 c 02, T Verhoeff Oneindig–11 Oneindige getallen Algebra¨ısch oneindig bestaat niet (in volle glorie) ∞−1=x • x niet eindig, want eindig plus 1 Solutions workout exercises of the course signal processing basics 5esa0 version january 12, 15 chapter and exercise 2πf 2π and 10 ω0 now, find the location
gamesguru 85 2 e^x (hyperbolic functions included), sin x, cos x, tan x all have a factorial in their power series The only useful examples I can think of that don't have a factorial are the inverse trig functions and the natural log Anyways, I don't want to get into an argument, I'll just rephrase myself, most power series that I've66 2 Definition Inverse Sine (also called arcsine) (2) y =sin−1x iff siny =x −1≤ x ≤ 1and −π 2 ≤ y ≤ π 2 −1 1 −π/2 π/2 Notice that the inverse sine appears to be differentiable everywhereMathmlcss Basic Examples Inline equation x Display equation x Fraction x 2 Binom (n k) Subcript and Superscripts x 2 y 3 a 1 2 Multiscripts BASE 1 2 3 4 5
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreFloatingpoint evaluation of the Hurwitz zeta function can be slow for complex arguments or highprecision numbers To increase the computational speed, you can reduce the floatingpoint precision by using the vpa and digits functions For more information, see Increase Speed by Reducing Precision The Hurwitz zeta function is related to other special functionsR h i h i P P R P h i 33 Statistical Mechanics I Re 07 Final Exam Review Problems The enclosed exams (and solutions) from the previous years are intended to help you




How To Sketch Polar Curves Krista King Math Online Math Tutor



New South Wales Higher School Certificate Mathematics Extension 2
FOURIER SERIES LINKSf(x) = (Πx)/2 x= 0 to 2Π Deduce Π/4 = 1 1/3 1/5 1/7 https//youtube/32Q0tMddoRwf(x) =x(2Πx) x= 0 to 2Π Show exam statistical physics (tn2622) tuesday, 31 october 06 the exam consists of three questions prepare each question on separate sheet and identified withFree function amplitude calculator find amplitude of periodic functions stepbystep
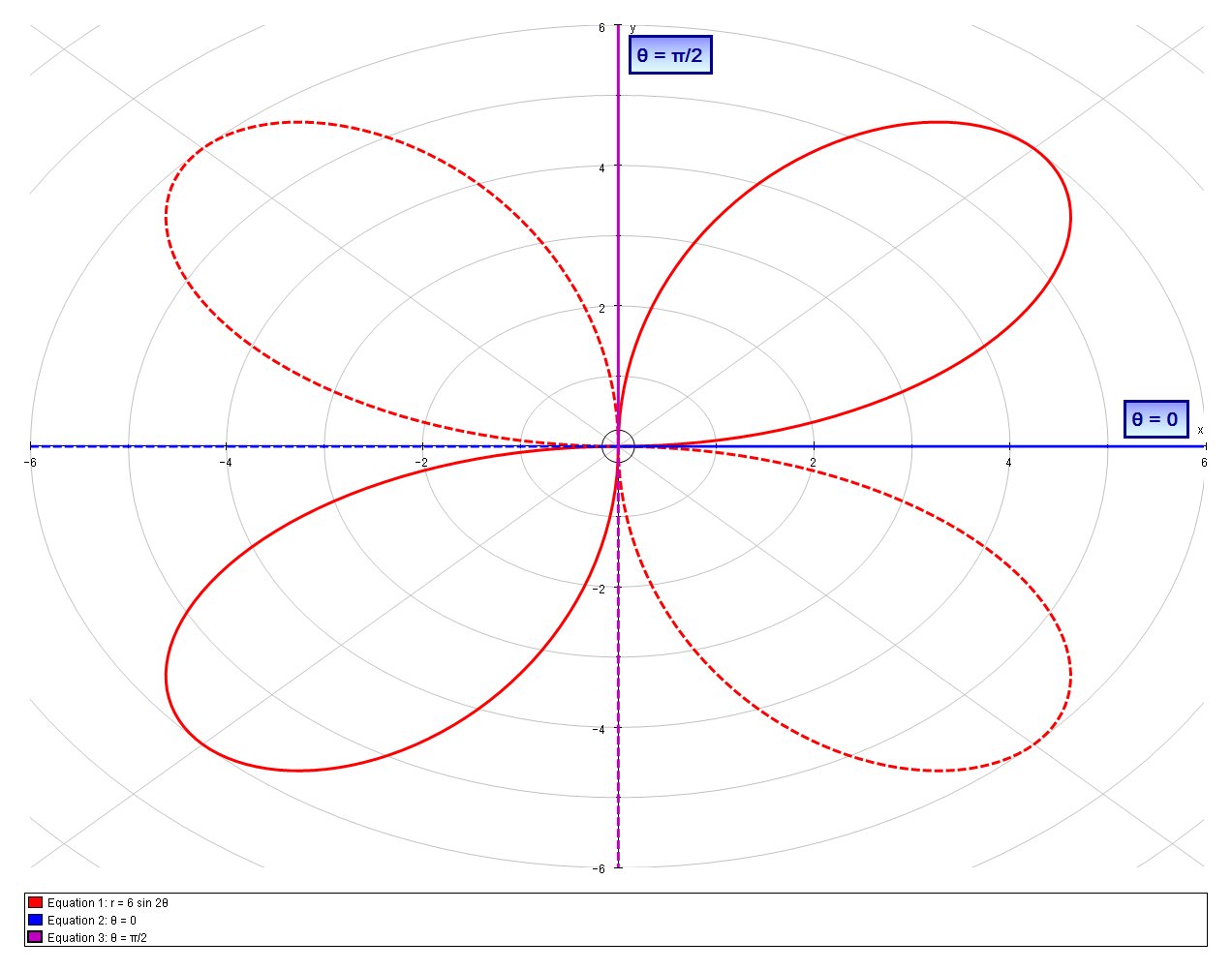



How Do You Find The Area Of One Petal Of R 6sin2theta Socratic




Evaluate The Following Limit Lim X Pi 4 Sqrt 2 Cos X Sin X 4x Pi 2
F = symsum(f,k) returns the indefinite sum (antidifference) of the series f with respect to the summation index kThe f argument defines the series such that the indefinite sum F satisfies the relation F(k1) F(k) = f(k)If you do not specify k, symsum uses the variable determined by symvar as the summation index If f is a constant, then the default variable is x为什么全体自然数平方的倒数和等于π^2/6? 全体自然数的平方的倒数和等于多少? 这是著名的 巴塞尔问题 。 现有的对这个问题的解答方法有很多,但在当时这个问题刚刚被提出的时候却难倒了一众数学家。 直到 的出现才第一次解决了这个问题,所以这个The following is a list of significant formulae involving the mathematical constant πThe list contains only formulae whose significance is established either in the article on the formula itself, the article Pi, or the article Approximations of π



Solved Problems
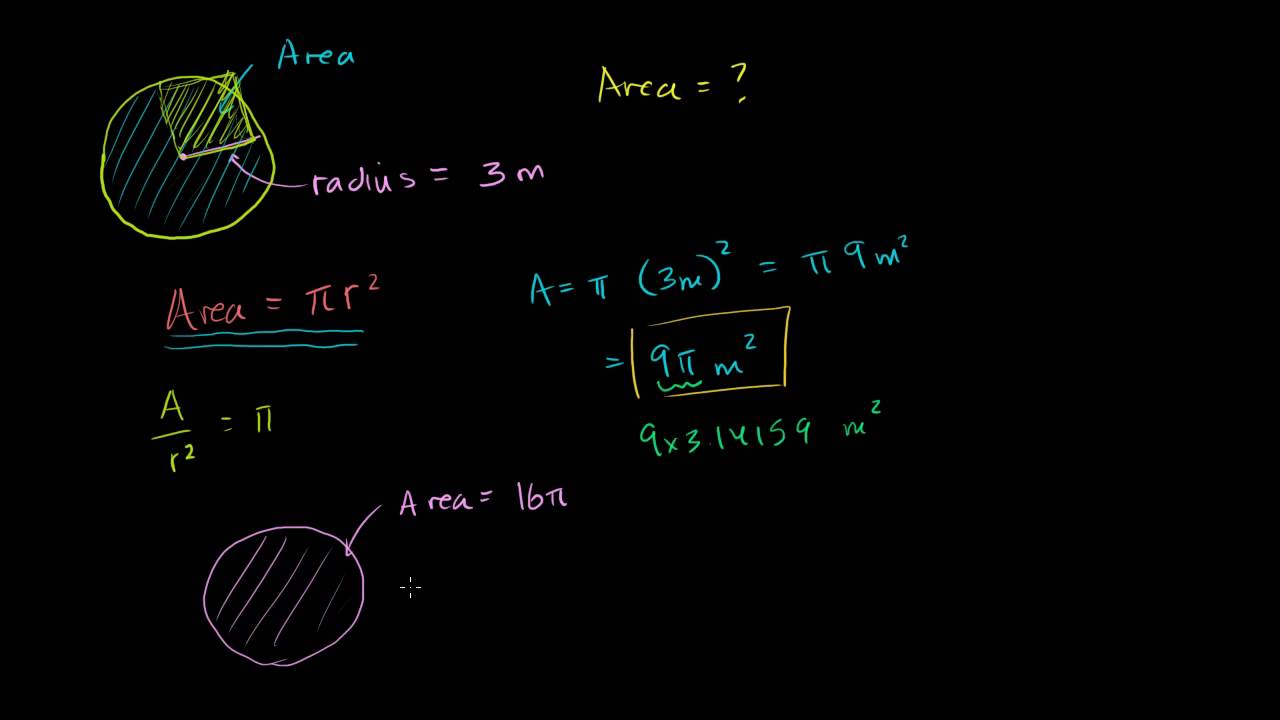



Area Of A Circle Web Formulas
2 Here the constant ω, with units of inverse time, is related to the period of oscillation T by ω = 2π/T See explanation This will be a long answer So what you want to find is int cos^6(x)dx There's a rule of thumb that you can remember whenever you need to integrate an even power of the cosine function, you need to use the identity cos^2(x) = (1cos(2x))/2 First we split up the cosines int cos^2(x)*cos^2(x)*cos^2(x) dx Now we can replace every cos^2(x) with the identity Issuu is a digital publishing platform that makes it simple to publish magazines, catalogs, newspapers, books, and more online Easily share your publications and get




Tinkutara Equation Editor Math Forum Question




10 Ft62 3 10 18 P 2 P 2 22 Gauthmath
Solution for 6) Σ n ) Π 2" Social Science AnthropologyLetting fk ˘ Pk n˘1 cn(x¡x0)n, we have fk â f on B(x0;†) because f is real analytic on B(x0;†) Also, by Theorem 81, f 0 k ˘ Pk n˘1 ncn(x¡x0)n¡1 is such that {f 0 k} converges uniformly to f 0 on B(x0;†) (this means the radius of convergence of f 0 k is some number R0 ‚ †)We're going to prove that fk/(x¡x0) has the same radius of convergence as f 0 k, and that willTheorem A For each point c in function's domain lim x→c sinx = sinc, lim x→c cosx = cosc, lim x→c tanx = tanc, lim x→c cotx = cotc, lim x→c cscx = cscc, lim



Webpage Of Dr Tom Cuchta




Pi Day 21 Quotes Wishes Messages Greetings And Hd Images
75 FMore proofs that π2/6 = P∞ n=1 1/n 2 370 76 FRiemann's remarkable ζfunction, probability, and π2/6 373 77 FSome of the most beautiful formulæ in the world IV 3 Chapter 8 Infinite continued fractions 3 81 Introduction to continued fractions 390 FSome of the most beautiful formulæ in the world V 394D ℓ Figuur 1 Het naaldenexperiment van Buffon rig op het blad vallen Dan kan de naald snijdend zijn met een lijn van het blad of niet Als je deze proef nu vele malen herhaalt en de verhouding bijhoudt tussenhet aantal keer dat jeFree math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor
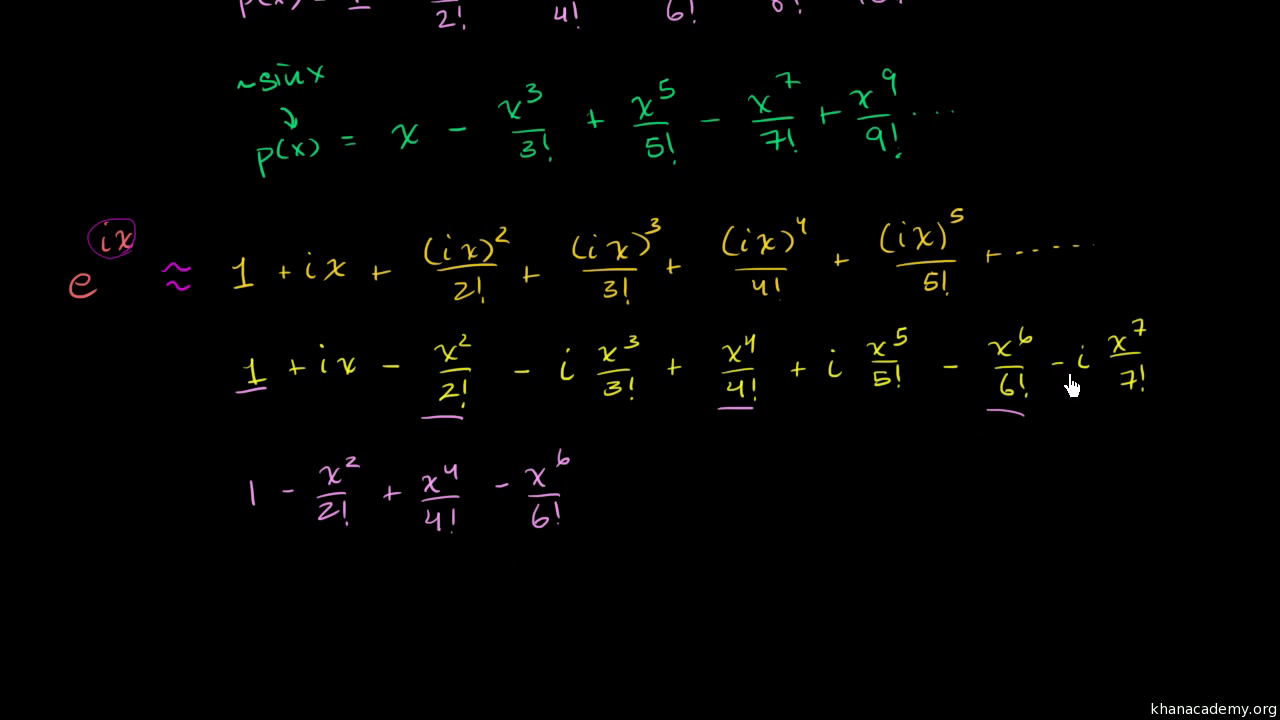



Euler S Formula Euler S Identity Video Khan Academy




Conditionals And Loops
4 Chapter 10 Techniques of Integration EXAMPLE 1012 Evaluate Z sin6 xdx Use sin2 x = (1 − cos(2x))/2 to rewrite the function Z sin6 xdx = Z (sin2 x)3 dx = Z (1− cos2x)3 8 dx = 1 8 Z 1−3cos2x3cos2 2x− cos3 2xdx Now we have four integrals to evaluate Z 1dx = x and Z ガンマ関数を \(2\) 階微分、及び \(3\) 階微分して \(\displaystyle x=1,\frac{1}{2}\) としたときの値は以下のようになります。グラムシュミットの正規直交化法 一般の n n n 次元ベクトル空間で通用する話ですが,ここでは高校生でも馴染みのある空間ベクトル( n = 3 n=3 n = 3 の場合)で説明します。 三次元の場合をしっかり理解すれば一般の場合の理解も容易です。




Taylor Series Of Sin X At Pi 2 Math Calculus Taylor Series Showme
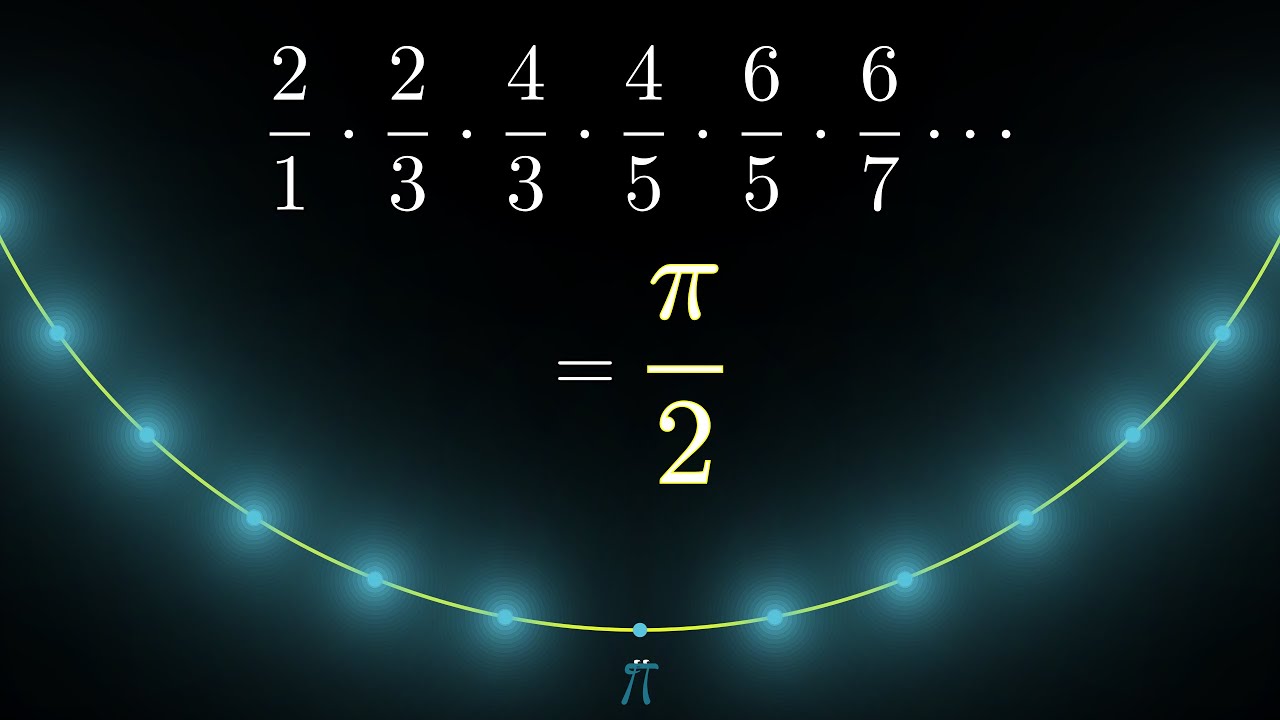



The Wallis Product For Pi Proved Geometrically Youtube
©G A0R1z2e 2K Ou TtIa I USto Sf DtYwxaDrFeo uL FLRCr C X gAMlslS PrziTg9hptOsg RrCe5s ae Br5v 2evd3P 3 bM ra pd ieW owmiYtyh D bI Pn Ff0i un Qint xex WGGe3oWmVeFtvrJy Hl Worksheet by Kuta Software LLC2 1 mω x 2 (11) 2m;Another proof that ζ(2) = π2/6 via double integration Tim Jameson (Slightly modified version of Math Gazette 97 (13), note 9744) Over the years several proofs that ζ(2) = X∞ n=1 1 n2 = π2 6 using just double integrals and elementary calculus have appeared Perhaps the following
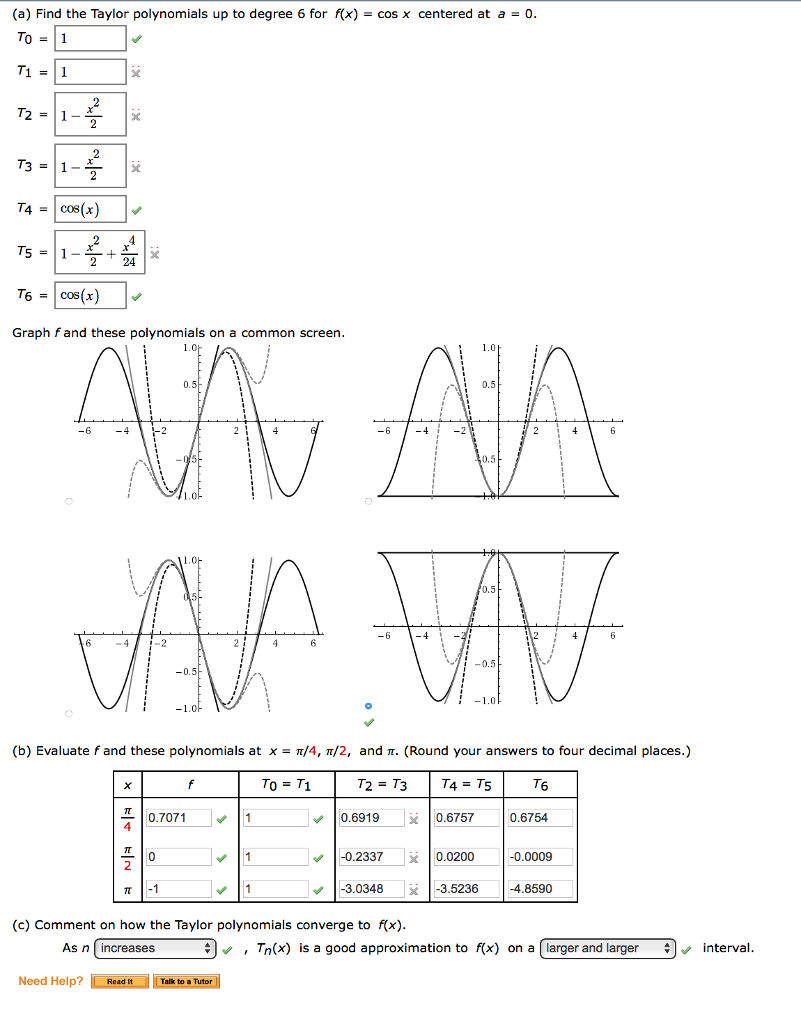



Evaluate F And These Polynomials At X Pi 4 Pi 2 And Chegg Com
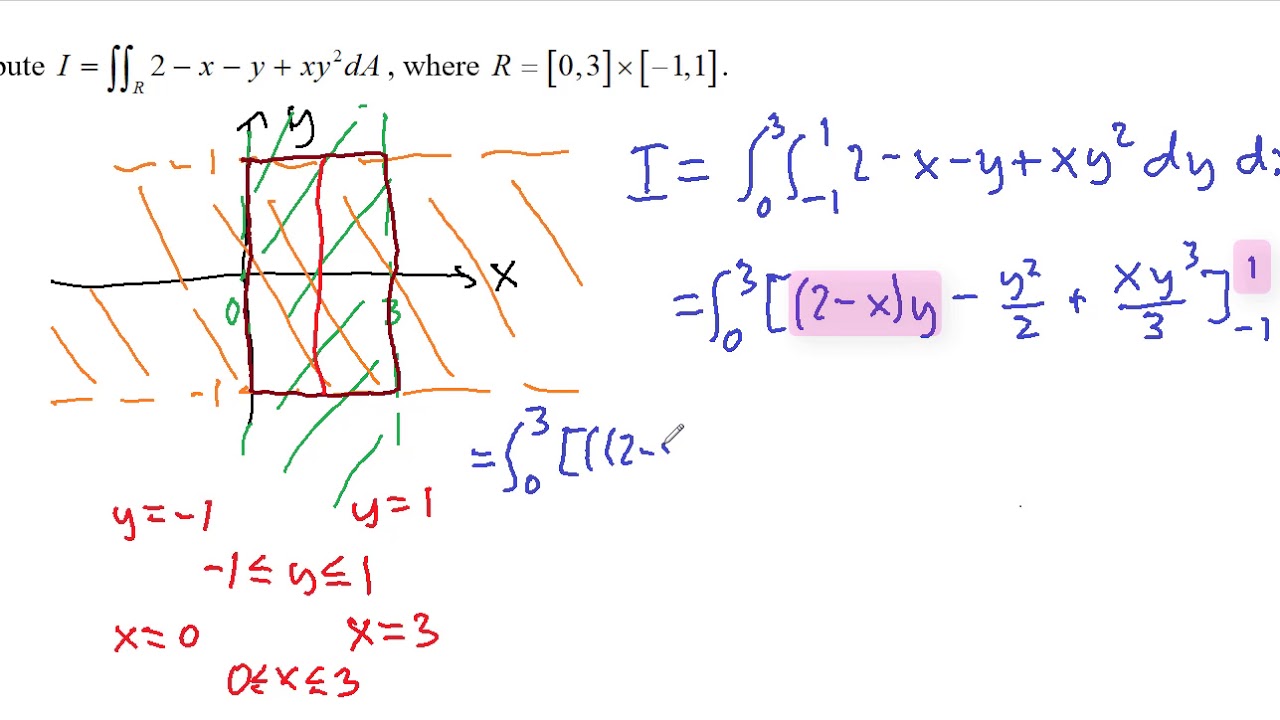



Double Integrals Volume And Average Value
An Infinite Sequence Representation of Pi 0 1 2 ∗32 2 3 ∗42 π2 − 9 3 Proof The above sequence can also be expressed as j=1 ∞ j − 1 j Hj 1L2 This canHet gebied G wordt ingesloten door de grafiek van f en de lijnen x=p , y=c en y=d G wordt gewenteld om de lijn x=p Dan geldt er dat I x = a G =π∙ c d f inv y p 2 dy Dit is te begrijpen door de translatie T(p, 0) uit te voeren f gaat dan over in de functie g gegeven door g x =f(xp) en het gebied G gaat dan over in een gebied G * We moeten dan G * wentelen om de y asI PMAT Question Paper IPM Indore Quantitative Ability Solve questions from IPMAT Question Paper from IPM Indore and check the solutions to get adequate practice The best way to ace IPMAT is by solving IPMAT Question Paper
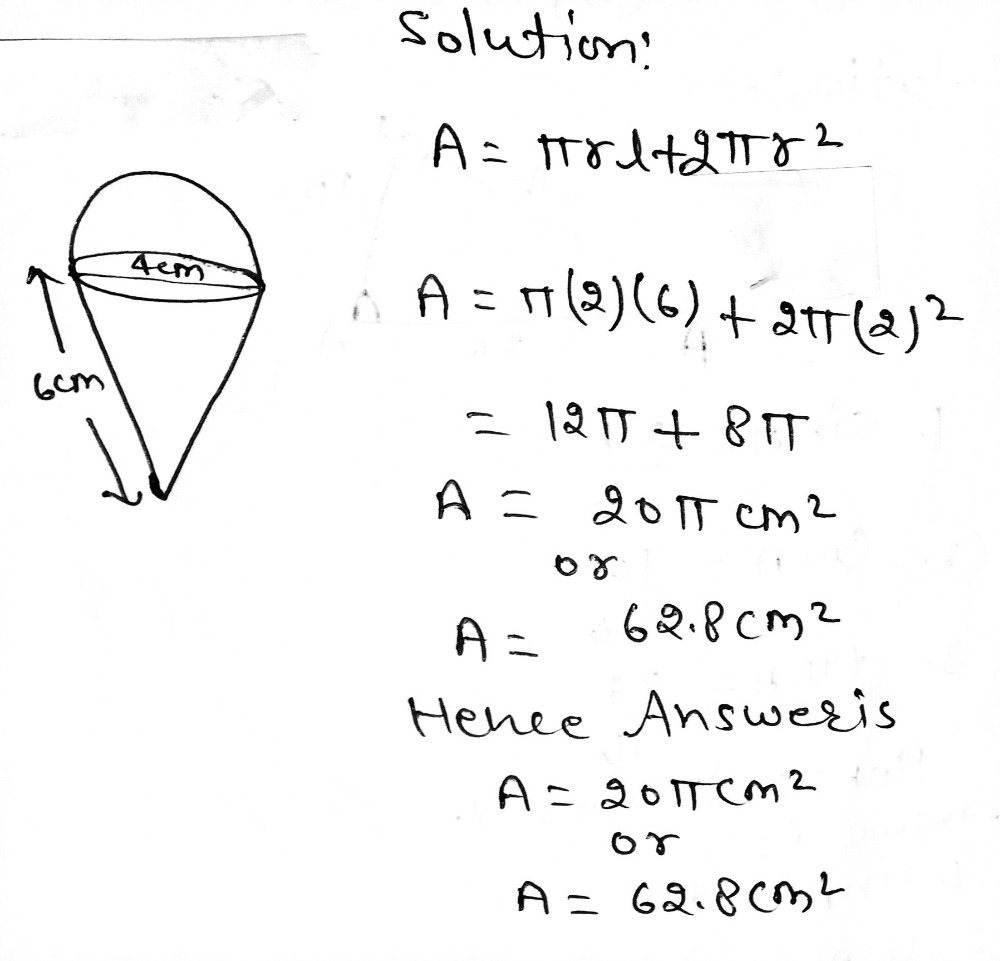



2 6 Points The Ice Cream Cone Below Consi Gauthmath




Wifi Password Integral Answer Math Online Tom Circle
The Basel problem is a problem in mathematical analysis with relevance to number theory, first posed by Pietro Mengoli in 1650 and solved by Leonhard Euler in 1734, and read on 5 December 1735 in The Saint Petersburg Academy of Sciences Since the problem had withstood the attacks of the leading mathematicians of the day, Euler's solution brought him immediate fame when he was(53) A bosonic gas is known to have a power law density of states g(ε) = Aεσ per unit volume, where σ is a real number (a) Experimentalists measure Tc as a function of the number density n and make a loglog plot of their results They find a beautiful straight line with slope 3 7 That is, Tc(n) ∝ n3/7Assuming the phase transition they observe is an ideal BoseEinstein condensation




Pi Day March 14 21 Events Activities History Exploratorium




The Early History Of Pi Pdf Free Download



Proving Int 0 Infty Mathrm E X 2 Dx Frac Sqrt Pi 2 Mathematics Stack Exchange




Pdf Another Elementary Proof Of Sum N Ge 1 1 N 2 Pi 2 6 And A Recurrence Formula For Zeta 2k
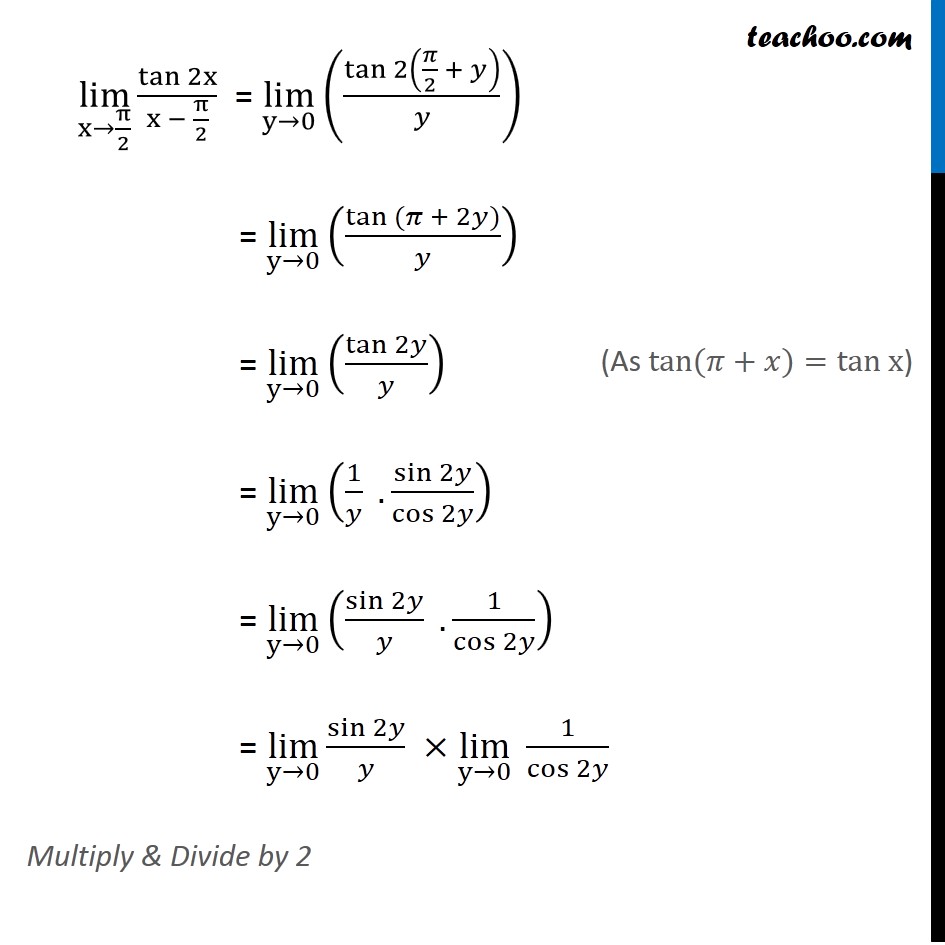



Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11




Math Polar Coordinates Ppt Download




What Is Pi And How Did It Originate Scientific American



Www Jstor Org Stable 10 4169 Math Mag 85 5 361
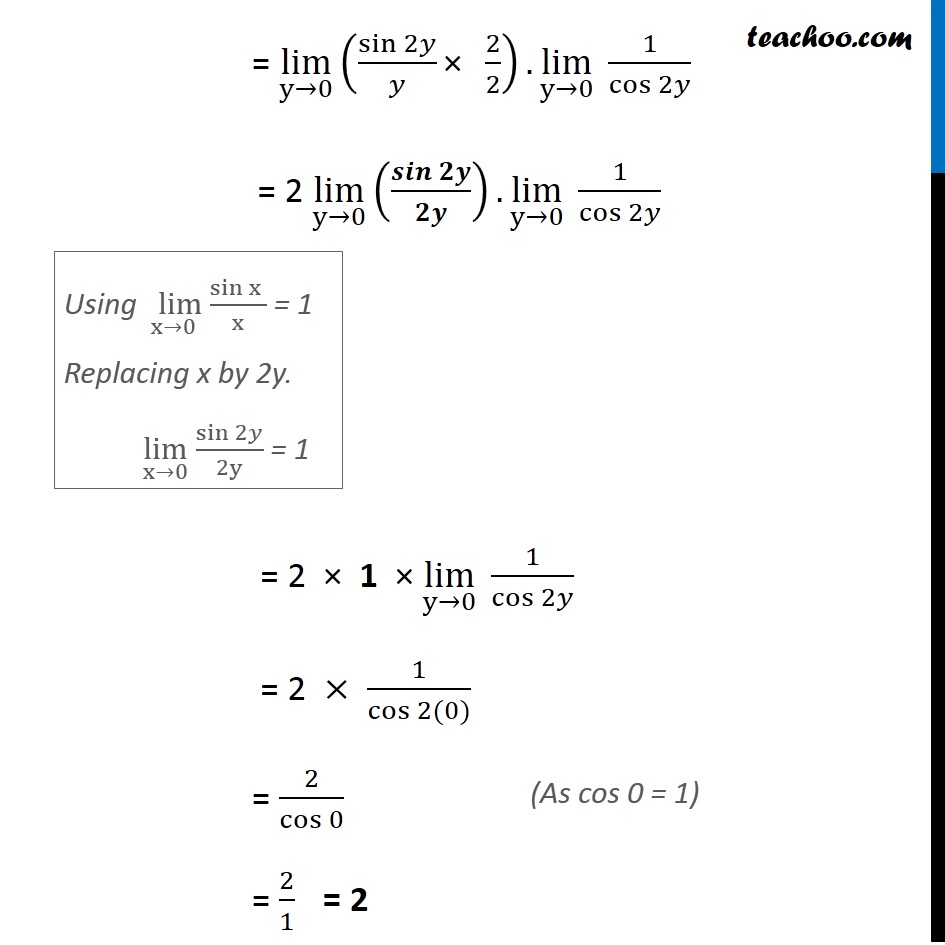



Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11
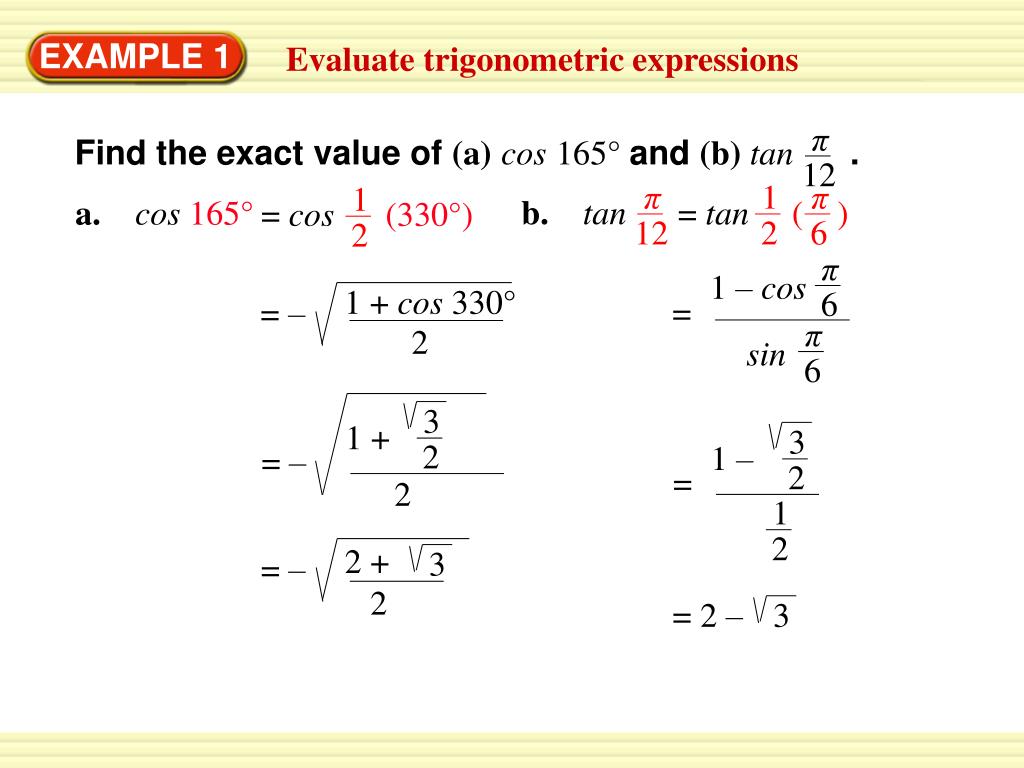



Ppt Example 1 Powerpoint Presentation Free Download Id
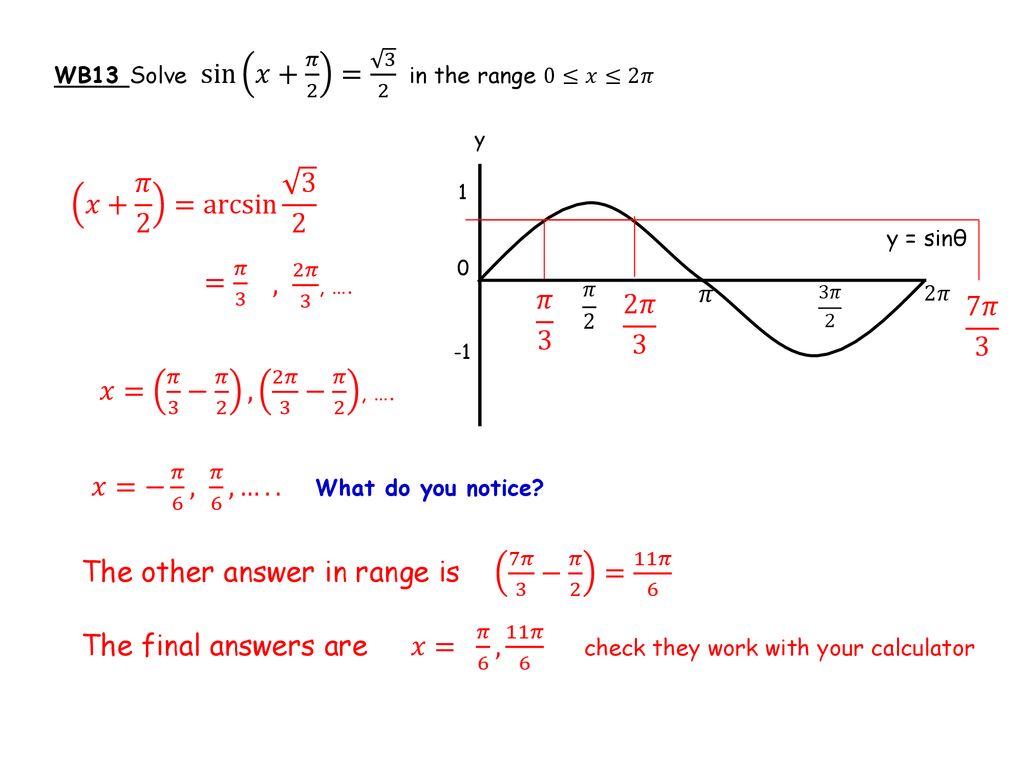



Trig Graphs And Equations Ppt Download




If 2 Sin 2 Pi 2 Cos 2 X 1 Cos Pi Sin 2x X Ne 2n 1 Pi 2 N In I Then Cos 2x Is Equal To




Int 0 Pi 2 Sqrt 1 Cos 2x Dx
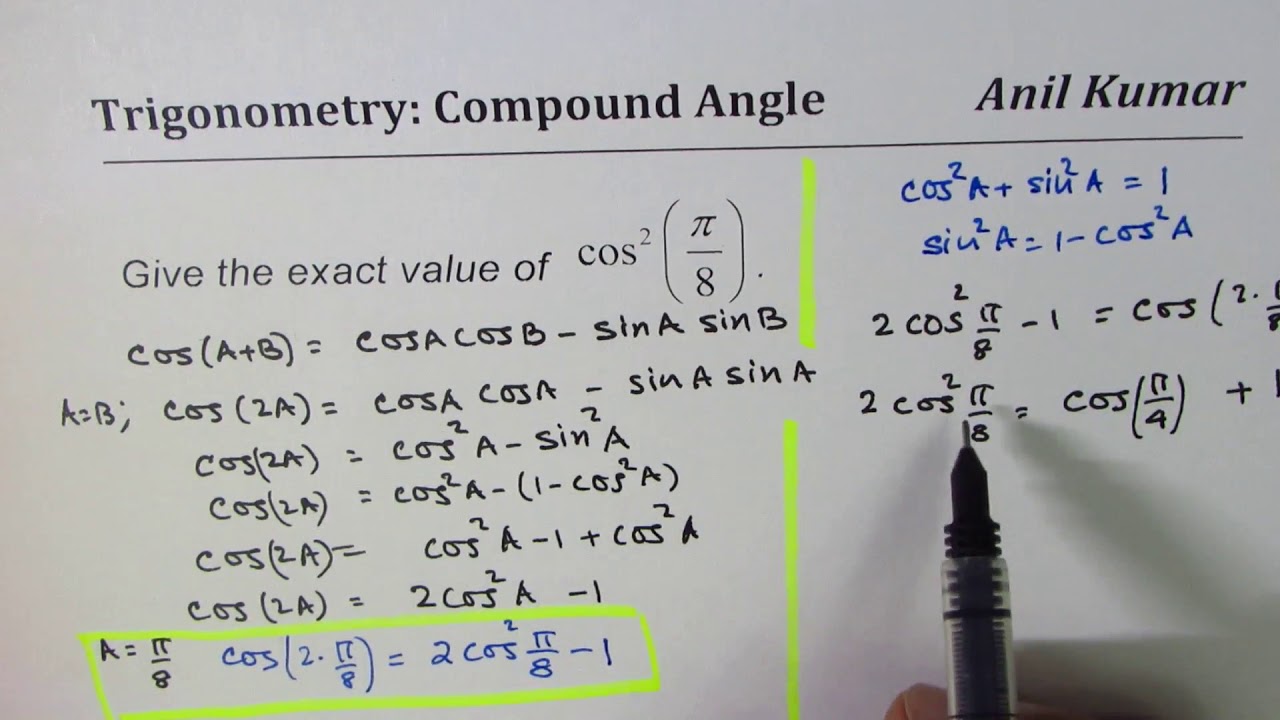



Find Exact Value Of Cos 2 Pi 8 Youtube




And H 2 6 Meam Ngares 1 00 L 9 7 Calculat Gauthmath




Relating Circumference And Area Video Khan Academy




Calculate The Value Of Tan Pi 2 Pi 6 Brainly In




Ex 13 1 22 Lim X Pi 2 Tan 2x X Pi 2 Chapter 13 Class 11
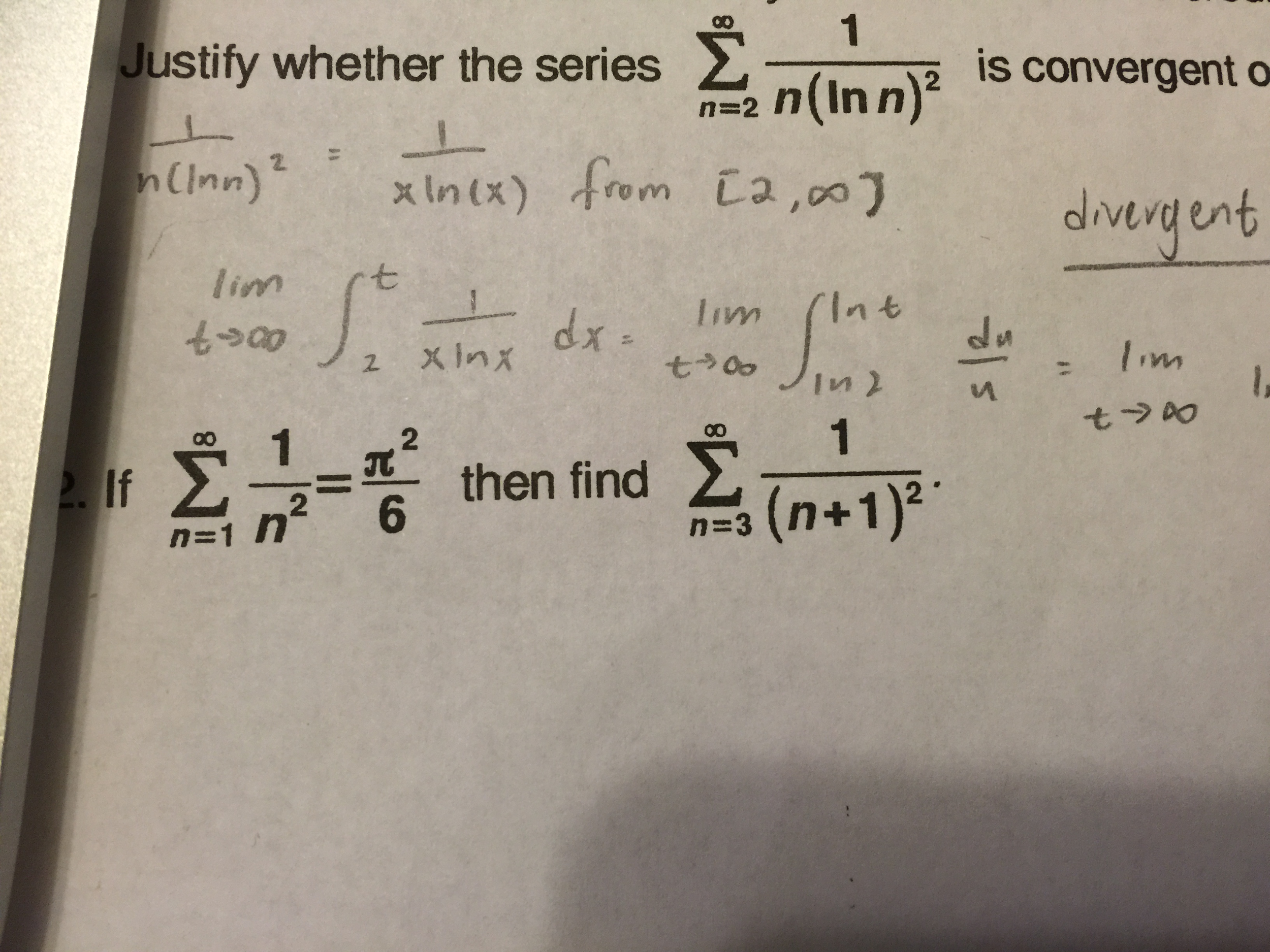



If Sigma Infinity N 1 1 N 2 Pi 2 6 Then Find Chegg Com



Home Hvl No Ansatte Tct Ftp V hydrodynamikk Matlab Ch03ny Eksempler Pdf
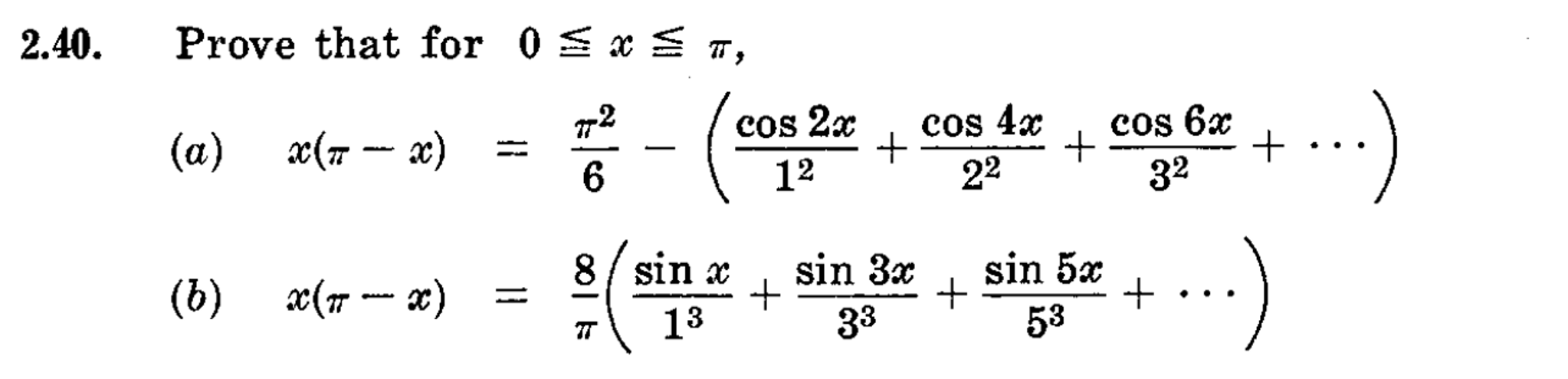



Prove That For 0 Lessthanorequalto X Chegg Com




Pdf Another Proof Of Z 2 P2 6 Using Double Integrals



1




How Do You Find The Equation Of The Tangent Line To The Curve Y 2xsinx At Pi 2 Pi Socratic



1




Using The Pythagorean Trig Identity Video Khan Academy
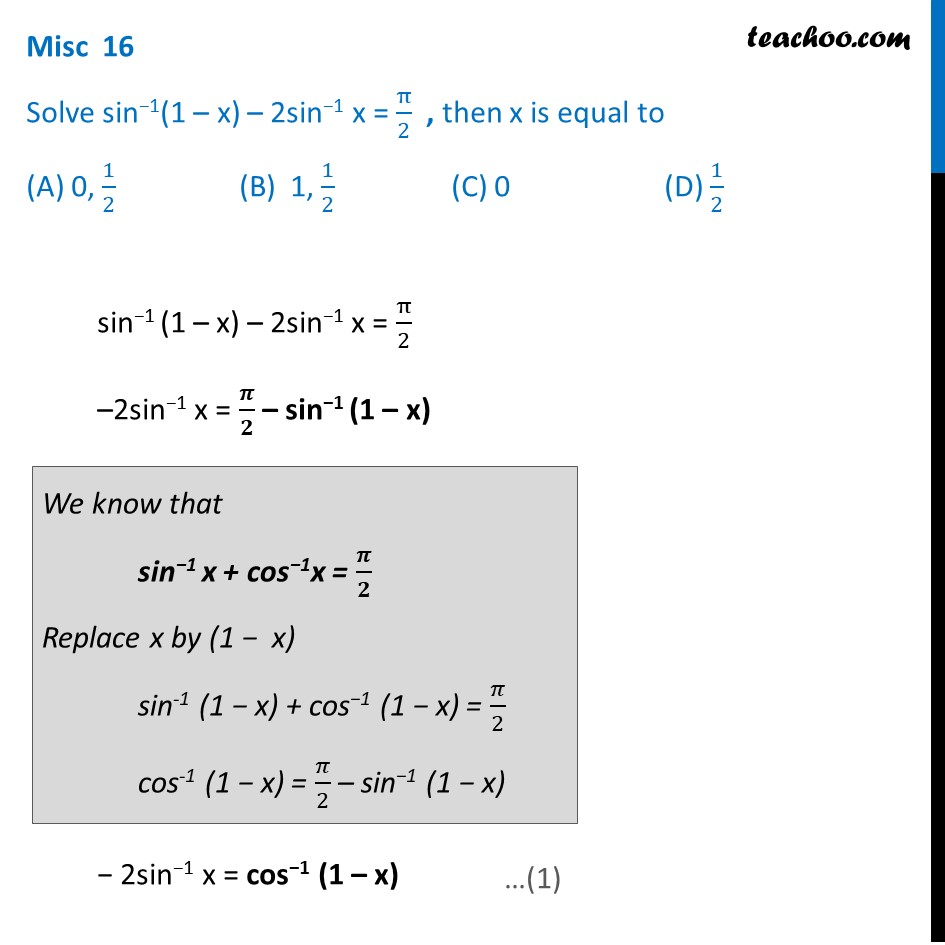



Solve Sin 1 1 X 2 Sin 1 X Pi 2 Then X Is Trigonometry
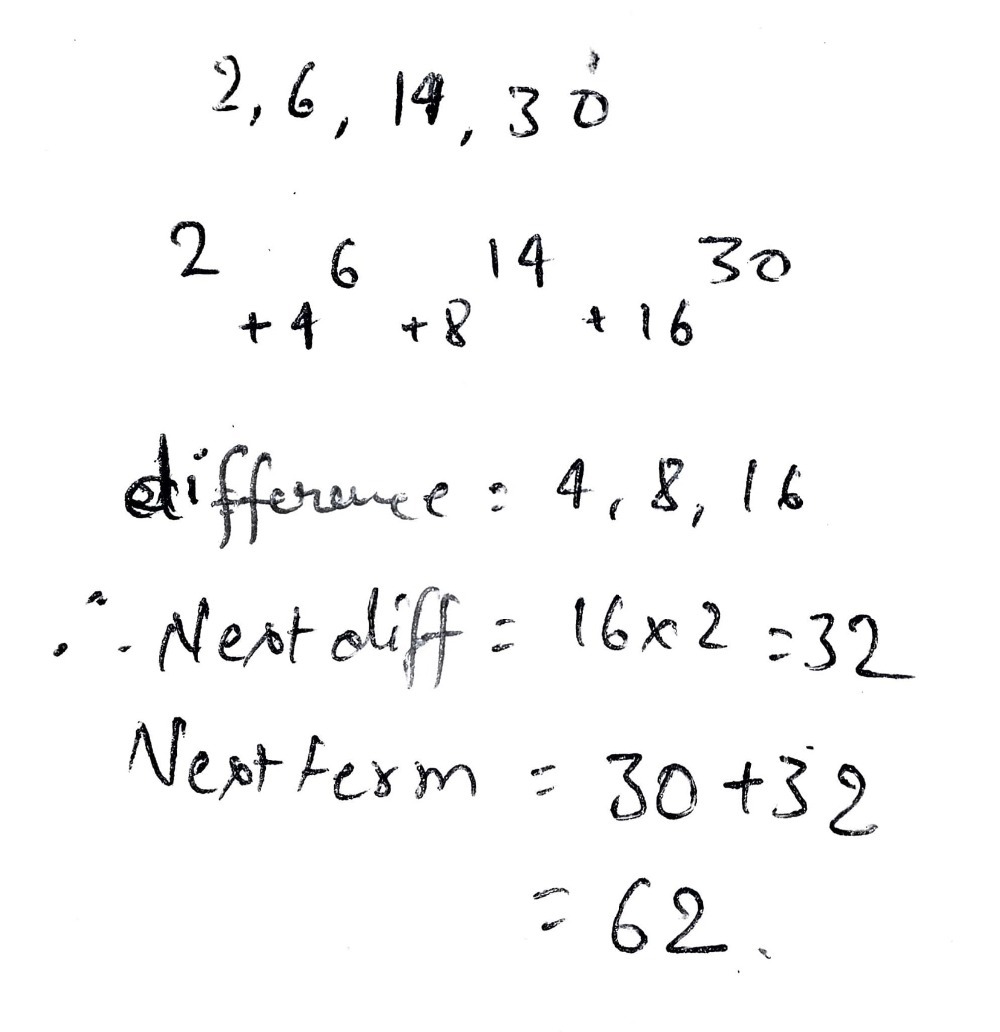



Question 12 Of 36 What Is The Next Ter Gauthmath




Sum Of 1 N 2 Youtube
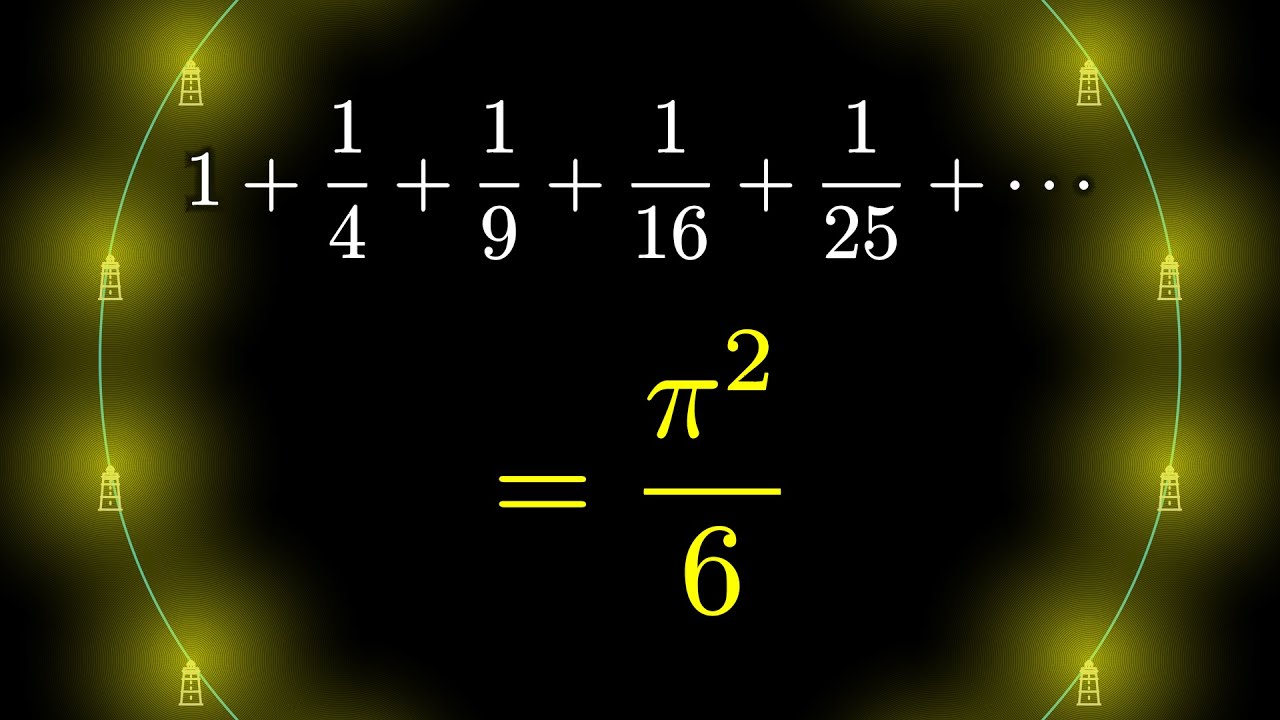



Why Is Pi Here And Why Is It Squared A Geometric Answer To The Basel Problem Youtube
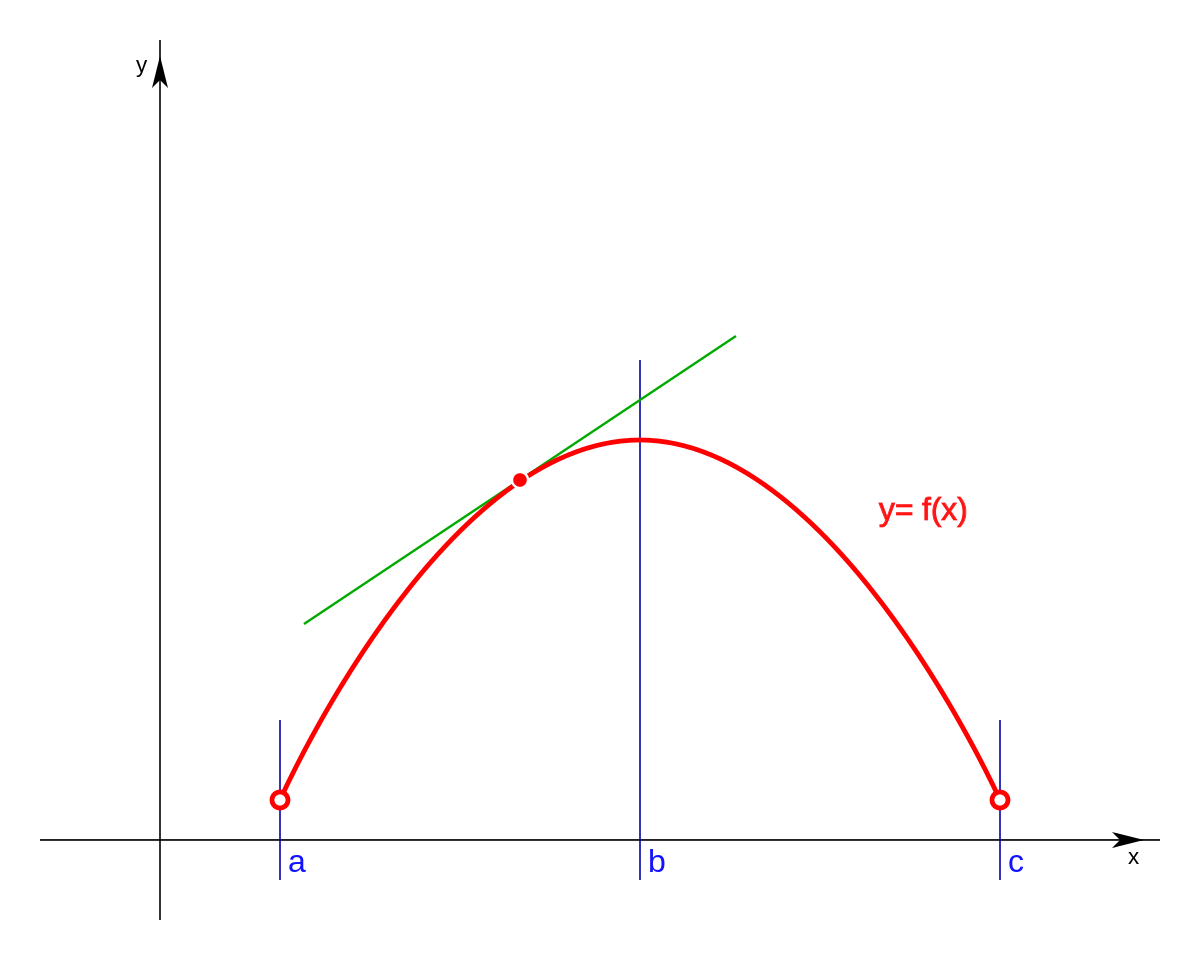



Slope Of A Curve At A Point Calculus Socratic
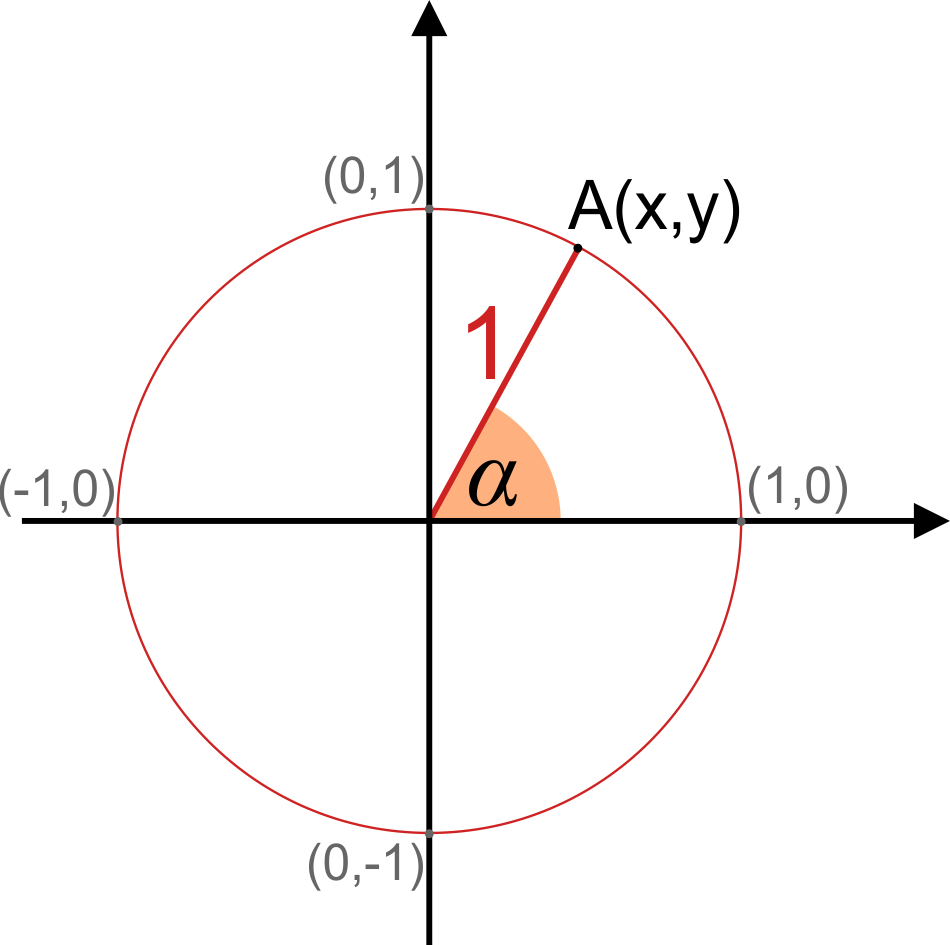



Unit Circle Calculator Find Sin Cos Tan



Tinkutara Equation Editor Math Forum Question 433




Angles In Radian Measure Iheartgeo




9 8 Powers And Roots Of Complex Numbers Ppt Download




Conditionals And Loops




1 Cos X Y Underline 2 Cos X Y Underline 3 Cos Gauthmath




Solution Evaluate The Integral Of Sin 6 Xdx From 0 To P 2




Fermat S Library New Paper A Proof That Euler Missed Solving The Basel Problem The Easy Way 1 1 4 1 9 1 16 P 2 6 Proof Here T Co Ew5uid3imh T Co Lcmxulqevh




Solve The Trignometric Equation 2 Sin 3x Pi 4 Sqrt 1 8 Sin2x Cos 2x Mathematics Stack Exchange




A Oeis
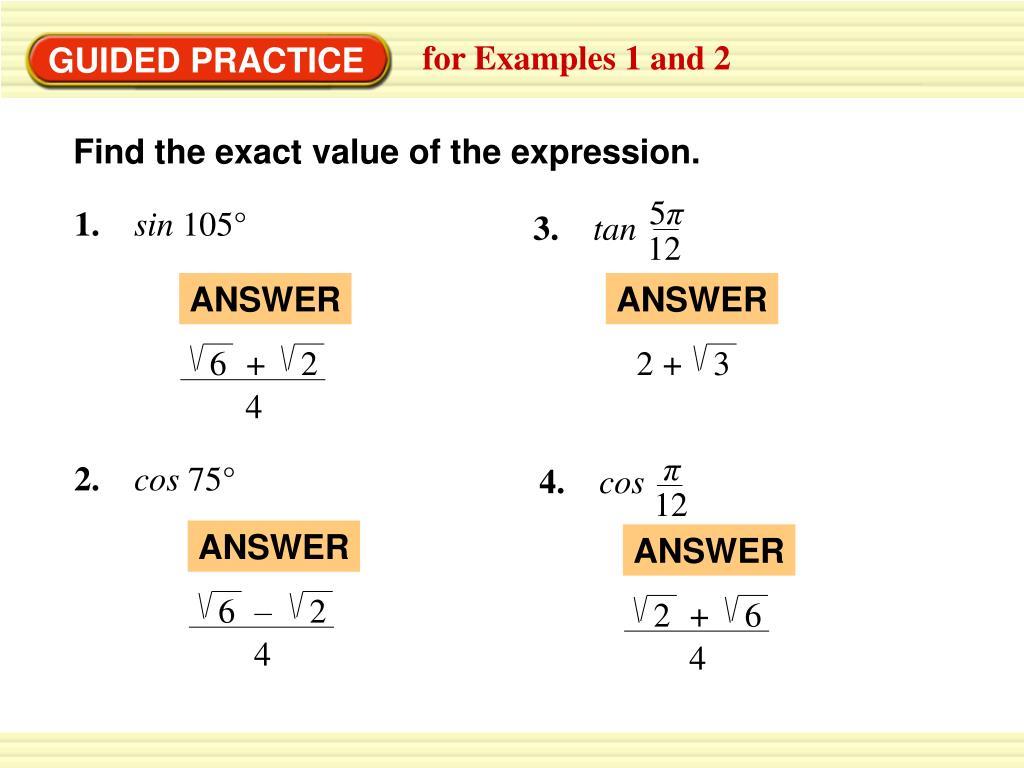



Ppt Example 1 Powerpoint Presentation Free Download Id




Ppt Lesson 14 1 For Use With Pages 908 914 Powerpoint Presentation Id
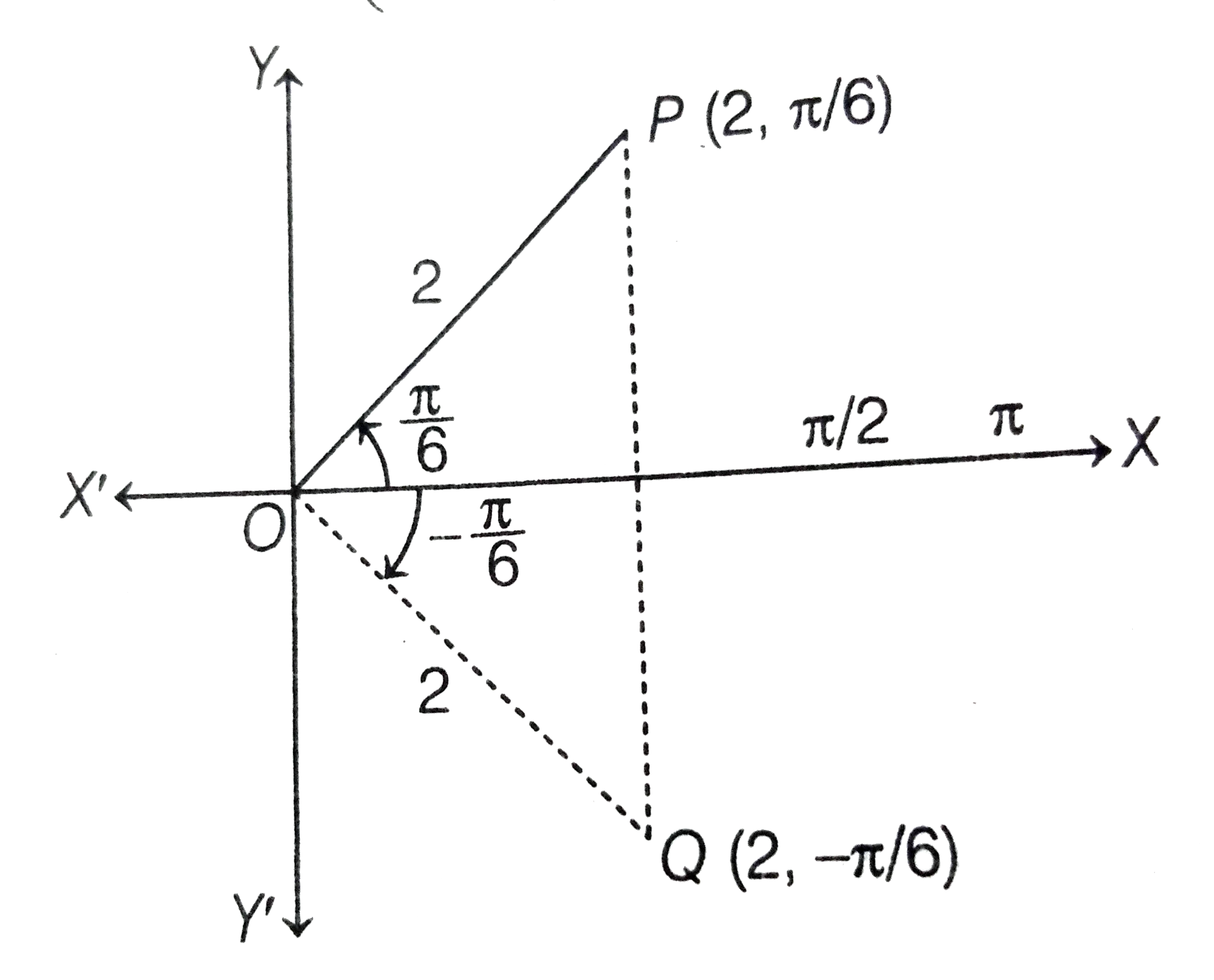



The Polar Co Ordinates Of P Are 2 Pi 6 If Q Is The Ima




Arcsin X Arccos X Pi 6 Inverse Trigonometric Equation Youtube




In The Given Fig If Abcd Is A Square The Value Of X Is 1 45 Circ 2 60 Circ Quad 3 70 Circ Quad 4 36 Circ Pi 2 A X




These 27 The Maximum Value Of Sin Left Theta Frac Pi 6 Right Cos Left Theta Frac Pi 6 7 Right Attained At Theta In 0 Pi 2 1 Frac Pi 18 2 Frac Pi 6



Pdf Another Simple Proof Of 1 1 2 2 1 3 2 P 2 6




B Consider The Series 1 P 4 P 2 4 2 2 P 3 Chegg Com
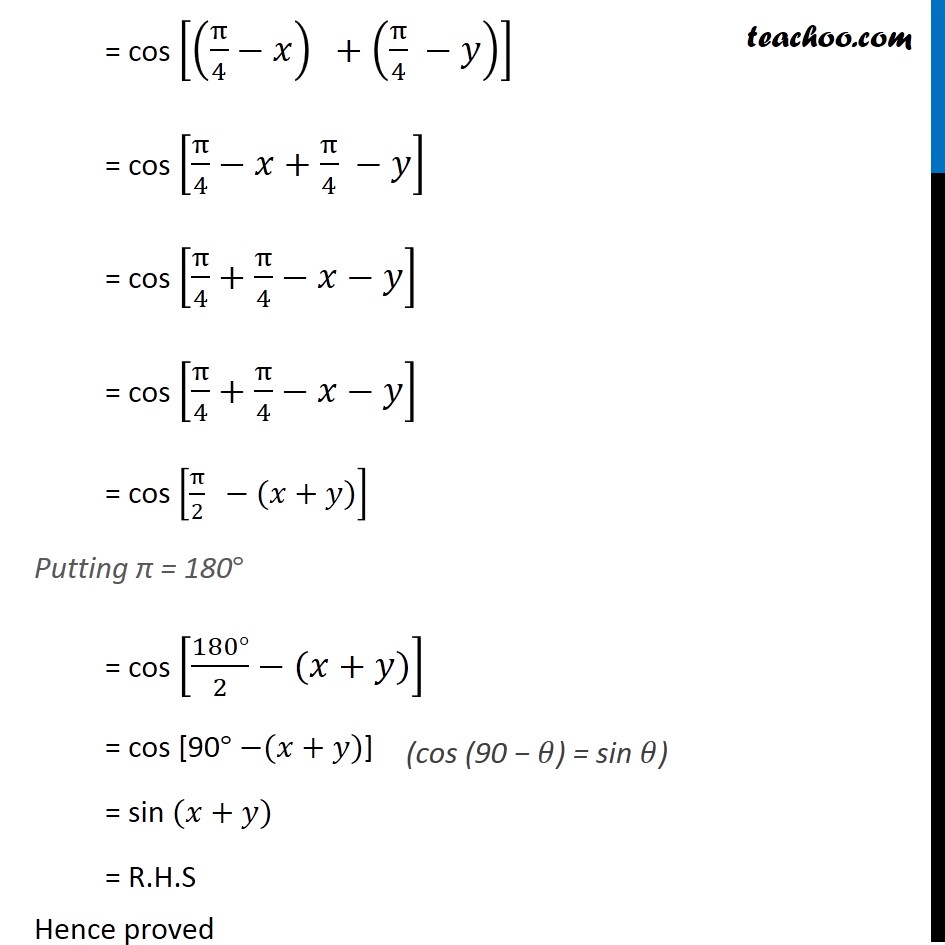



Ex 3 3 6 Prove That Cos Pi 4 X Cos Pi 4 Y Chapter 3
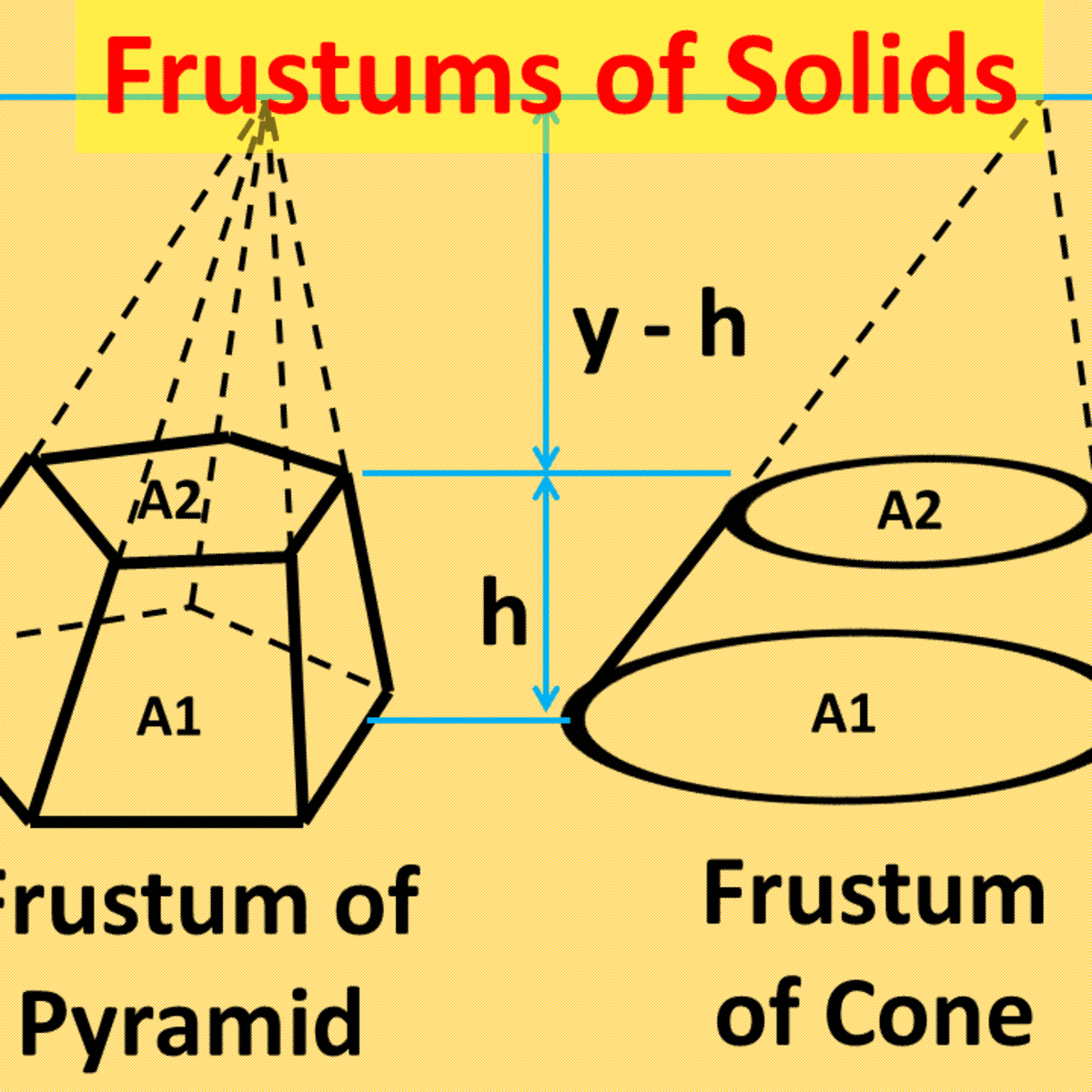



Finding The Surface Area And Volume Of Frustums Of A Pyramid And Cone Owlcation
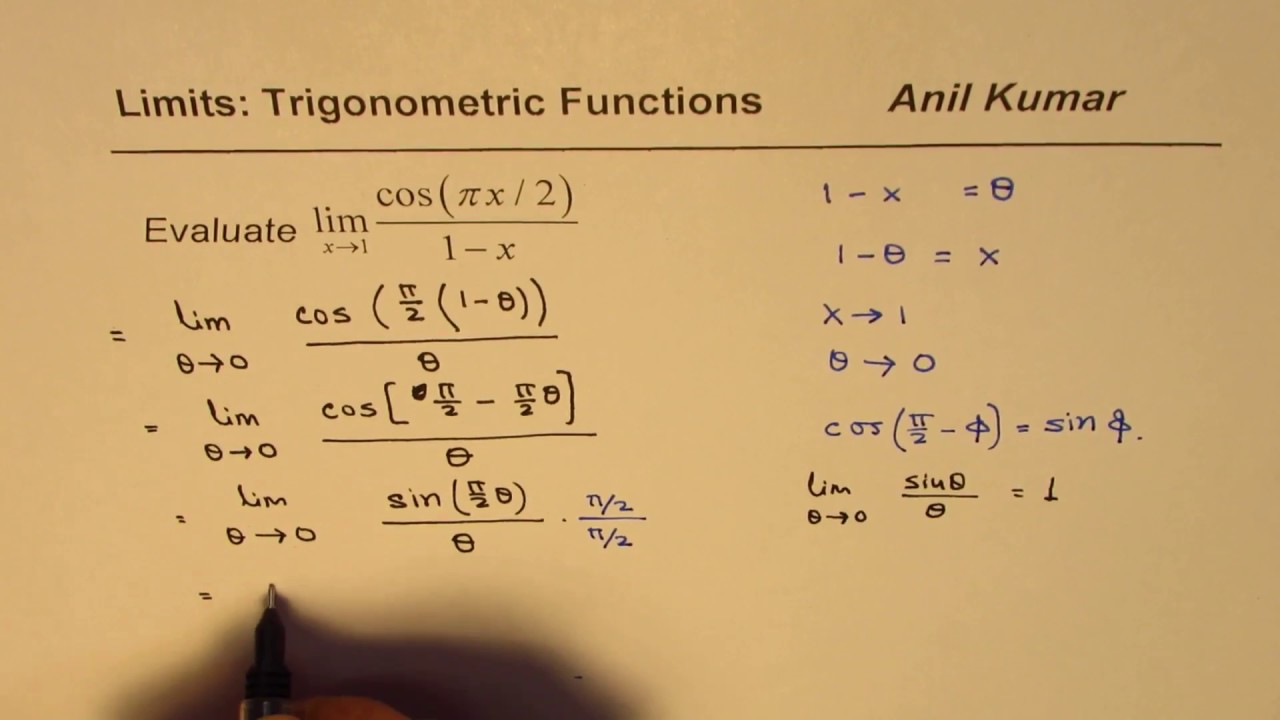



Limit Trigonometric Function Cos Pi X 2 1 X By Substitution Youtube




97 44 Another Proof That Z 2 P2 6 Via Double Integration The Mathematical Gazette Cambridge Core




Prove That Int 0 Pi 2 Sinx Sinx Cos X D Dx Pi 4




Double Integrals Volume And Average Value
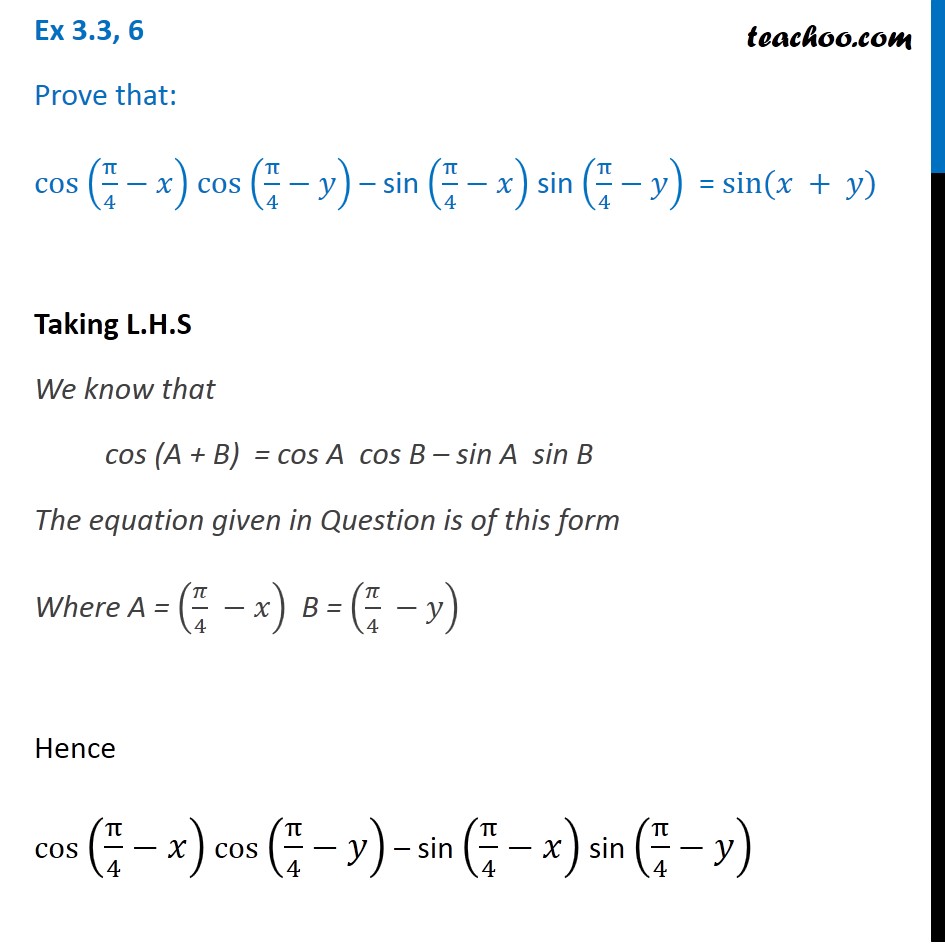



Ex 3 3 6 Prove That Cos Pi 4 X Cos Pi 4 Y Chapter 3




Cosmic Neutrino Background Ppt Download




Review A Vector Analysis Pdf Free Download
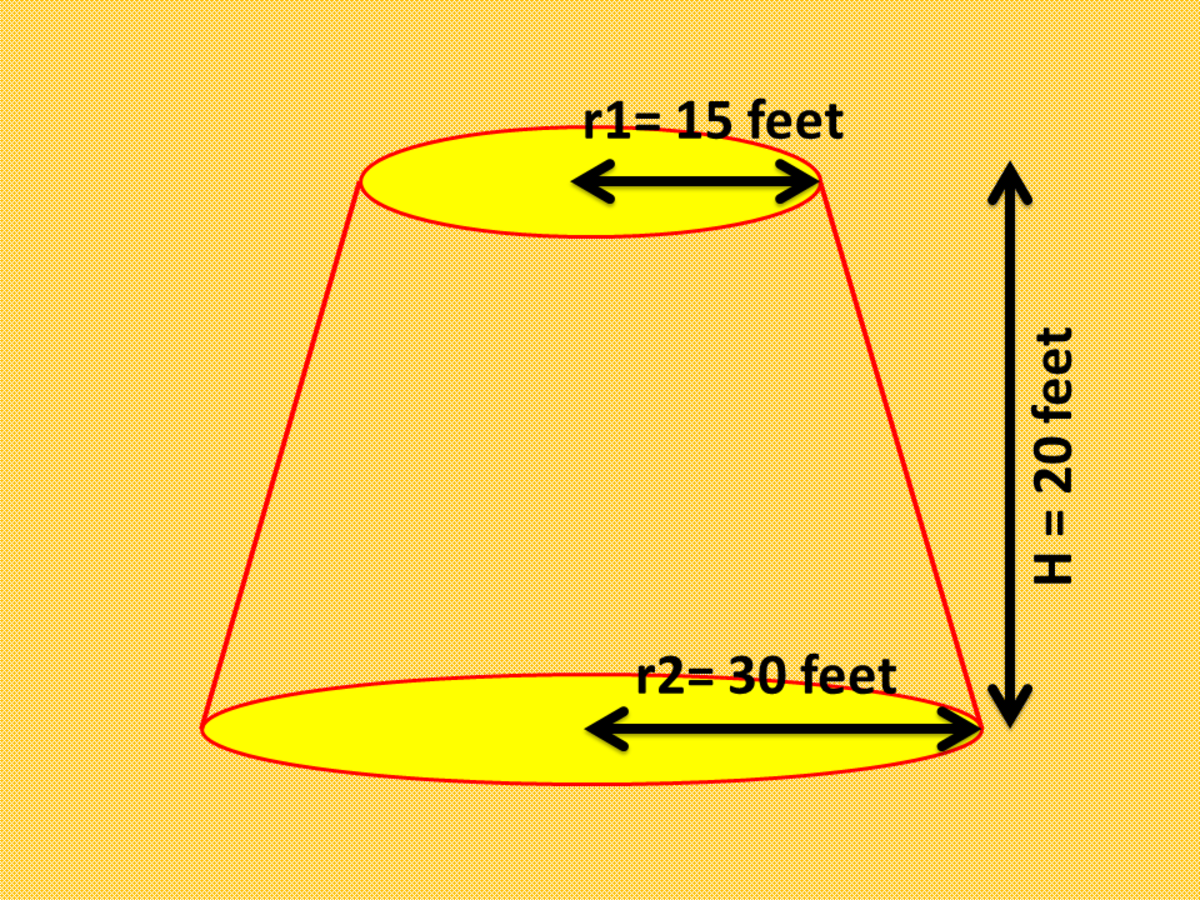



Finding The Surface Area And Volume Of Frustums Of A Pyramid And Cone Owlcation
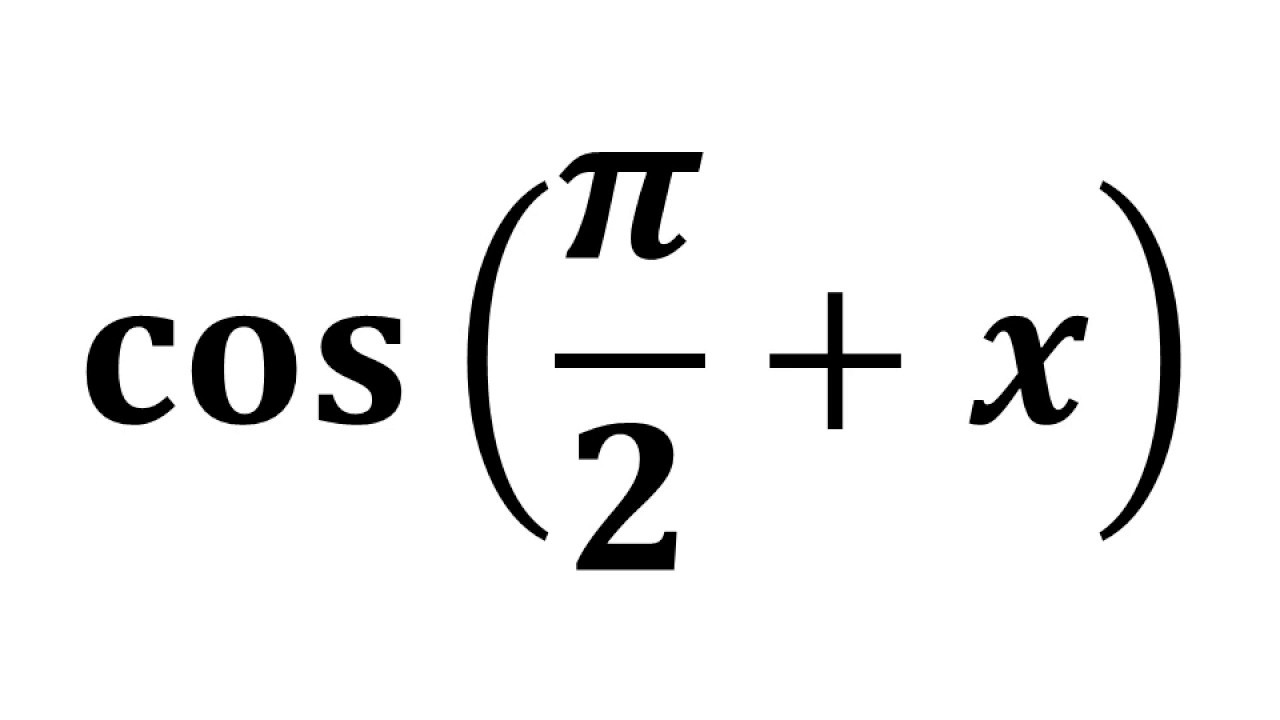



Cos Pi 2 X Cos Pi 2 Theta Youtube
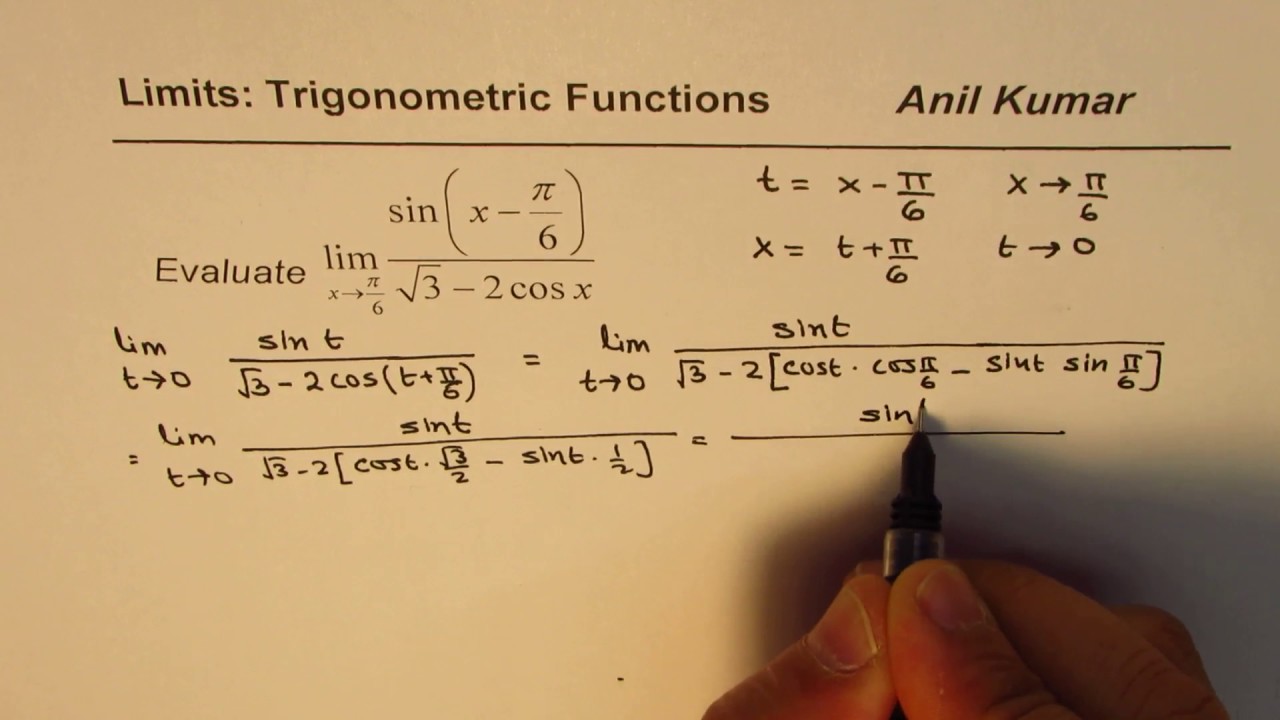



Limit Trigonometric Function Sin X Pi 6 Sqrt 3 2cosx Youtube




Use The Graph Below To Answer The Question That Follows Graph Of Curve That Passes Through The Brainly Com




2 2 Pi Cos Left Frac 2 Pi 2 64 1 Right Cos Left Frac 2 2 Pi 2 64 1 Right Ldots Ldots Cos Left Frac 2 64 Pi 2 64 1 Right 1 Frac 1 16
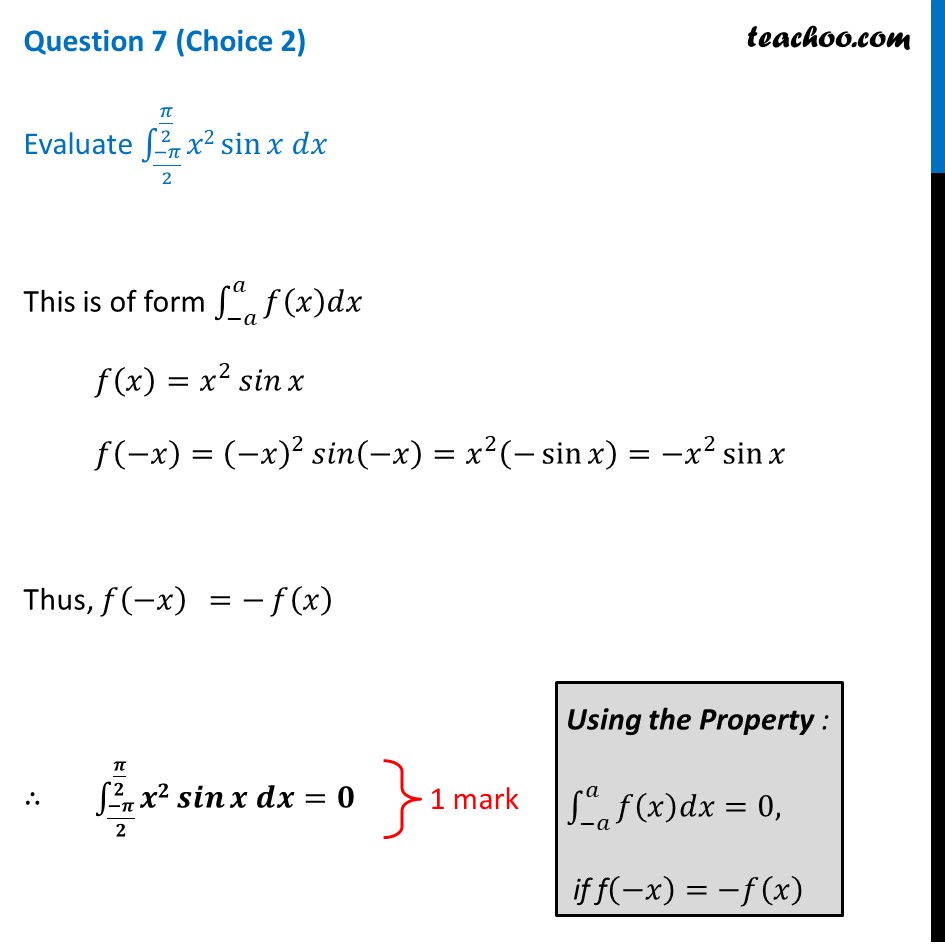



Evaluate Integral From P 2 To P 2 X 2 Sin Xdx Cbse Class 12 Sa




What Is The Volume Of The Solid Produced By Revolving F X Sinx X In 0 Pi Around The X Axis Socratic



1




James Tanton What S The Next Number In The Sequence 2 4 6 8 Write A Formula That Gives 2 For N 1 4 For N 2 6 For N 3 8 For




Proof 1 1 1 4 1 9 1 16 1 25 Pi 2 6 Youtube



Eem Eskisehir Edu Tr Cozzaim Eem 502 Icerik 5 Array Pdf



The Answer Is P 2 6 What S The Question Republic Of Mathematics Blog



Hapticity Wikipedia




For X In 0 Pi 2 Prove That Sin X Gt X X 2 6




Here Is Euler S Idea To Obtain The Formula 1 1 2 2 Chegg Com
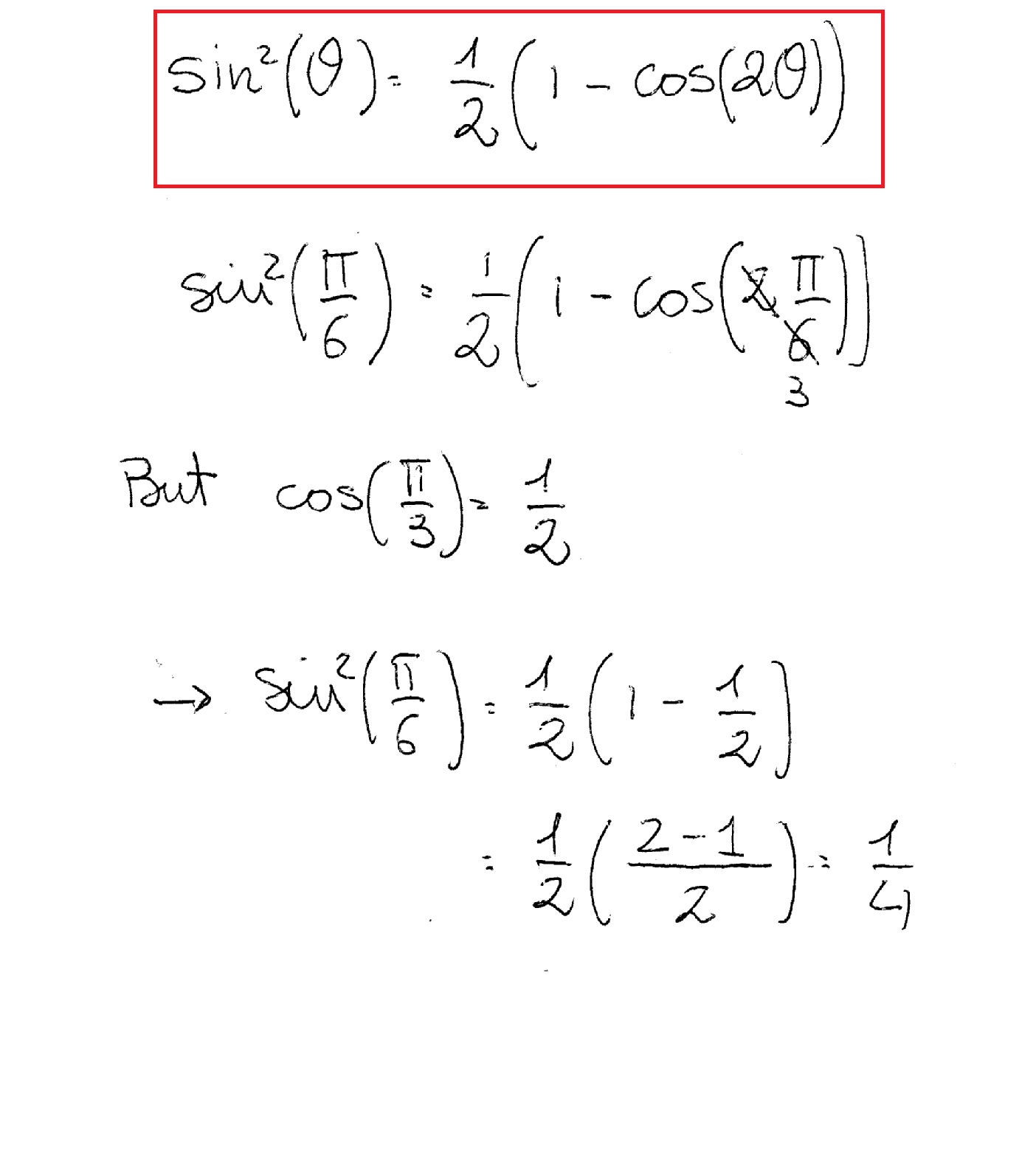



How Do You Use The Half Angle Formula To Simplify Sin 2 Pi 6 Socratic




1 Let F 1yz I 3xz J 9xy K Compute The Following A Div F F 1yz X B Curl F I J




Pi 2 62 2 Pi 3 2 Find Out




Symmetry Free Full Text Diagnosis Of The S P And S P Aromaticity By The Shape Of The Nicszz Scan Curves And Symmetry Based Selection Rules Html



1



Circular Functions Vce Methods




The Area Of The Region Formed By X 2 Y




1 38 Demostrar Ecuacion Sum 1 N 2 Pi 2 6 Youtube
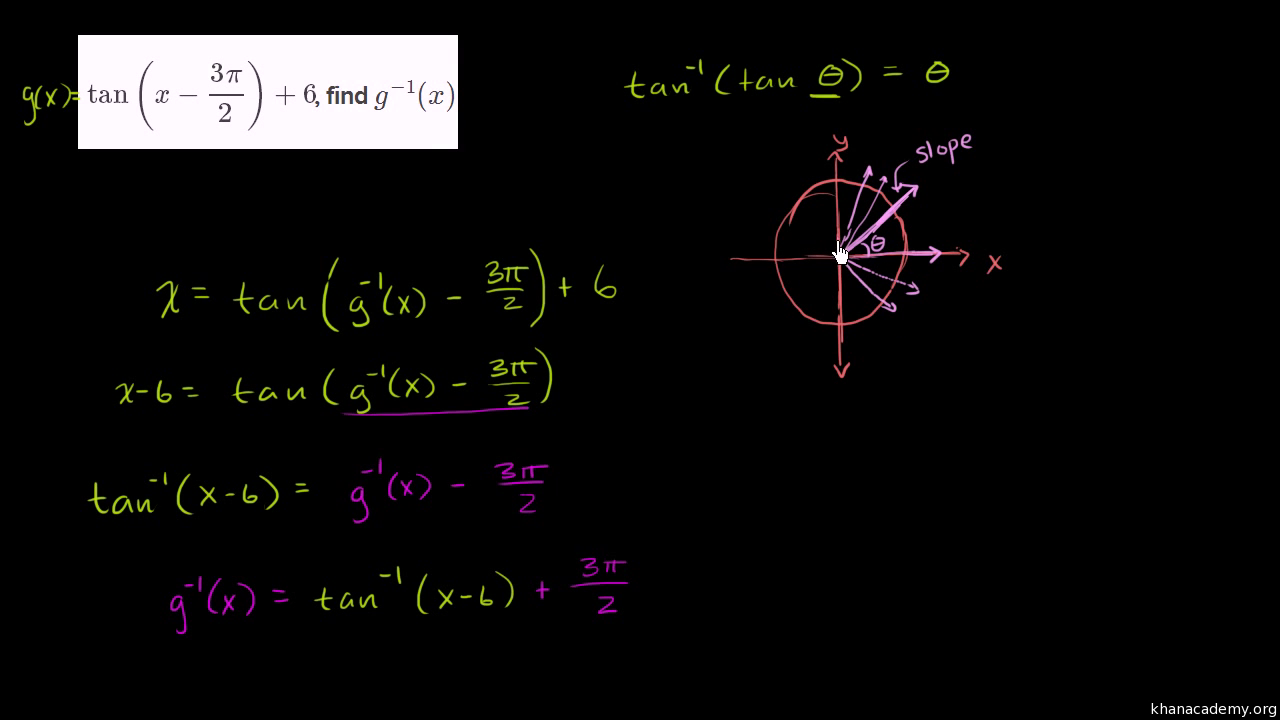



Domain Range Of Inverse Tangent Function Video Khan Academy
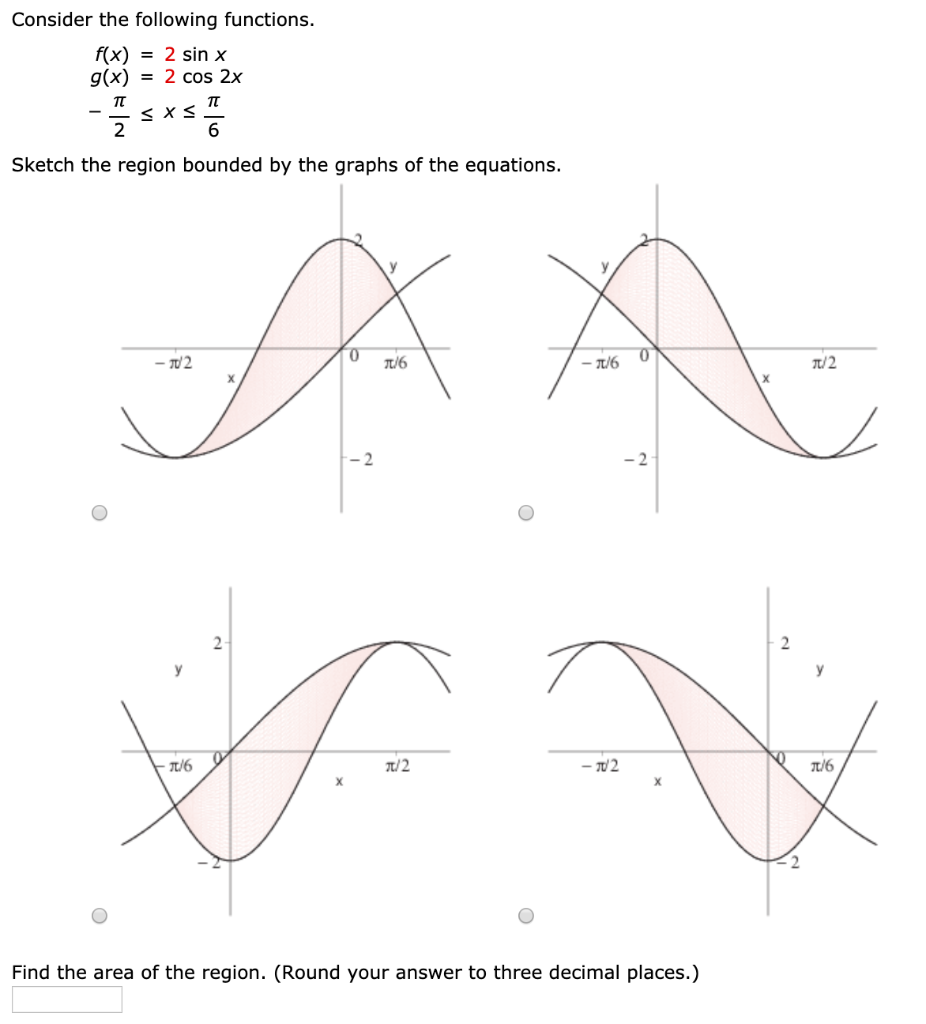



Consider The Following Functions F X 2 Chegg Com




But How Did Euler Do It A Beautiful Solution To The Famous Basel Problem Youtube




Pin On Math




S S 7 14 Q 2 Int 0 Infty Frac E X E X 1 Frac Pi 2 6 0space Org



0 件のコメント:
コメントを投稿